Advertisements
Advertisements
प्रश्न
If a sphere is inscribed in a cube, find the ratio of the volume of cube to the volume of the sphere.
उत्तर
In the given problem, we are given a sphere inscribed in a cube. So, here we need to find the ratio between the volume of a cube and volume of sphere. This means that the diameter of the sphere will be equal to the side of the cube. Let us take the diameter as d.
Here,
Volume of a cube (V1) = s3
= d3
Volume of a sphere (V2) `=(4/3)pi (d/2)^3`
`=(4/3) pi (d^3/8)`
`=(pid^3)/6`
Now, the ratio of the volume of sphere to the volume of the cube = `V_1/V_2`
`V_1/V_2 = d^3/(((pi d^3)/6))`
`= 6/pi`
So, the ratio of the volume of cube to the volume of the sphere is `6 : pi` .
APPEARS IN
संबंधित प्रश्न
Find the surface area of a sphere of diameter 14 cm .
A spherical ball of lead has been melted and made into identical smaller balls with radius equal to half the radius of the original one. How many such balls can be made?
How many balls each of radius 1 cm can be made by melting a bigger ball whose diameter is 8 cm?
What is the least number of solid metallic spheres, each of 6 cm diameter, that should be melted and recast to form a solid metal cone whose height is 45 cm and diameter 12 cm?
A sphere is placed inside a right circular cylinder so as to touch the top, base and lateral surface of the cylinder. If the radius of the sphere is r, then the volume of the cylinder is
Find the surface area and volume of sphere of the following radius. (π = 3.14 )
9 cm
The internal and external diameters of a hollow hemi-spherical vessel are 21 cm and 28 cm respectively. Find volume of material of the vessel.
The given figure shows the cross-section of a cone, a cylinder and a hemisphere all with the same diameter 10 cm and the other dimensions are as shown.
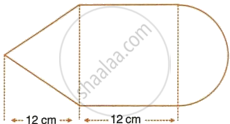
Calculate :
- the total surface area.
- the total volume of the solid and
- the density of the material if its total weight is 1.7 kg.
A spherical cannon ball, 28 cm in diameter is melted and recast into a right circular conical mould, the base of which is 35 cm in diameter. Find the height of the cone, correct to one place of decimal.
A manufacturing company prepares spherical ball bearings, each of radius 7 mm and mass 4 gm. These ball bearings are packed into boxes. Each box can have maximum of 2156 cm3 of ball bearings. Find the:
- maximum number of ball bearings that each box can have.
- mass of each box of ball bearings in kg.
(use π = `22/7`)
