Advertisements
Advertisements
प्रश्न
In below fig, determine the value of x.

उत्तर १
Since sum of all the angles round a point is equal to 360°.Therefore
⇒ 3x + 3x +150 + x = 360°
⇒ 7x° = 360° -150°
⇒ 7x = 210°
⇒ x = `210/7`
⇒ x = 30°
उत्तर २
In the given figure:
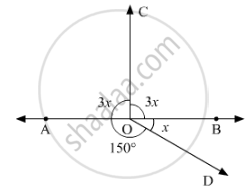
AOB is a straight line. Thus,∠AODand ∠BODform a linear pair.
Therefore their sum must be equal to180°.
We can say that
∠AOD+∠BOD = 180°
It is given that ∠AOD=150°, substituting this value in equation above, we get:
`150° + x = 180°`
` x = 180° - 150°`
` x =30°`
APPEARS IN
संबंधित प्रश्न
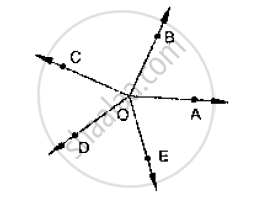
In the given below fig, rays OA, OB, OC, OP and 0E have the common end point O. Show
that ∠AOB + ∠BOC + ∠COD + ∠DOE + ∠EOA = 360°.
The supplement of an acute angle is .................
An angle is equal to its supplement. Determine its measure.
An angle is equal to five times its complement. Determine its measure.
State, true or false:
A line segment 4 cm long can have only 2000 points in it.
out of \[\overleftrightarrow{AB},\overrightarrow{AB},\overleftarrow{AB}\] and `overline(AB)` which one has a fixed length?
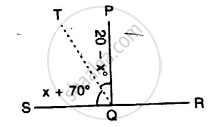
In the given figure, find ∠PQR.
Write the complement of (30 − a)°
Write the supplement of x°
AB, CD and EF are three lines intersecting at the same point.
(i) Find x, if y = 45° and z = 90°.
(ii) Find a, if x = 3a, y = 5x and r = 6x.