Advertisements
Advertisements
प्रश्न
In below fig, ray OS stand on a line POQ. Ray OR and ray OT are angle bisectors of ∠POS
and ∠SOQ respectively. If ∠POS = x, find ∠ROT.

उत्तर
Given,
Ray OS stand on a line POQ
Ray OR and Ray OT are angle bisectors of ÐPOS and ÐSOQ respectively
`∠`POS = x
`∠`POS and `∠`QOS is linear pair
ÐPOS + ÐQOS = 180°
x + ÐQOS = 180°
`∠`QOS = 180 - x
Now, ray or bisector `∠`POS
∴ `∠`ROS = `1/2` `∠`POS
= `1/2`× x [ ∵ `∠`POS = x]
`∠`ROS = x
Similarly ray OT bisector `∠`QOS
∵`∠`TOS = `1/2` `∠`QOS
= `180/2` - x [∵ `∠`QOS = 180 - x]
=` 90/2` - x
∴ `∠`ROT = `∠`ROS + `∠`ROT
= `x/2`+ 90 -`x/2`
= 90°
∴`∠`ROT = 90°
APPEARS IN
संबंधित प्रश्न
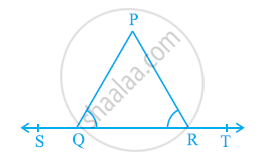
In the given figure, ∠PQR = ∠PRQ, then prove that ∠PQS = ∠PRT.
Write the complement of the following angles .
30°
If the supplement of an angle is three times its complement, find the angle.
An angle is 14° more than its complementary angle. What is its measure?
In the below Fig, OA and OB are opposite rays.
If y = 35°, what is the value of x?
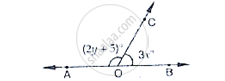
In the below fig, ACB is a line such that ∠DCA = 5x and ∠DCB = 4x. Find the value of x.
statement are true and false
Angles forming a linear pair are supplementary.
The opposite sides of a quadrilateral are parallel. If one angle of the quadrilateral is 60°,
find the other angles.
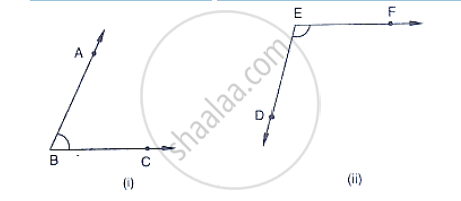
In the below fig, arms BA and BC of ∠ABC are respectively parallel to arms ED and EF of
∠DEF. Prove that ∠ABC + ∠DEF = 180°.
In the given figure, if l1 || l2, what is the value of x?

