Advertisements
Advertisements
प्रश्न
In the given figure, A is the midpoint of YZ and G is the centroid of the triangle XYZ. If the length of GA is 3 cm, find XA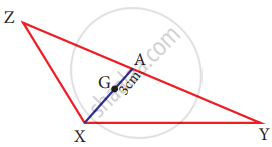
उत्तर
Given A is the midpoint of YZ.
∴ ZA = AY
G is the centroid of XYZ centroid divides each median in a ratio 2 : 1
⇒ XG : GA = 2 : 1
`"XG"/"GA" = 2/1`
`"XG"/3 = 2/1`
XG = 2 × 3
XG = 6 cm
XA = XG + GA
= 6 + 3
⇒ XA = 9 cm
APPEARS IN
संबंधित प्रश्न
In the given figure, point S is any point on side QR of ΔPQR Prove that: PQ + QR + RP > 2PS
Draw an obtuse angled Δ STV. Draw its medians and show the centroid.
Draw an obtuse angled Δ LMN. Draw its altitudes and denote the orthocentre by ‘O’.
Draw an isosceles triangle. Draw all of its medians and altitudes. Write your observation about their points of concurrence.
The centroid of a triangle divides each medians in the ratio _______
In any triangle the centroid and the incentre are located inside the triangle
The centroid, orthocentre, and incentre of a triangle are collinear
Identify the centroid of ∆PQR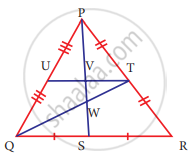
In ∆DEF, DN, EO, FM are medians and point P is the centroid. Find the following
If DO = 8, then FD = ?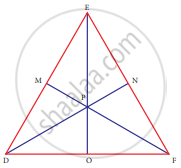
In ∆ABC, AD is the bisector of ∠A meeting BC at D, CF ⊥ AB and E is the mid-point of AC. Then median of the triangle is ______.
