Question

In the given figure, AC || DE || BF. If AC = 24 cm, EG = 8 cm, GB = 16 cm, BF = 30 cm.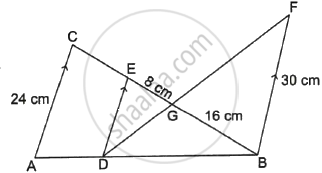

1. Prove ΔGED ∼ ΔGBF
2. Find DE
3. DB : AB

Answer 1

a. In ΔGED and ΔGBF,

DE || BF

`\implies` ∠GED = ∠GBF  ...(Alternate angles)

And ∠GDE = ∠GFB  ...(Alternate angles)

Also ∠DGE = ∠FGB  ...(Vertically opposite angles)

ΔGED ∼ ΔGBF   ...(AAA – axiom) 

Hence Proved

b. ΔGED ∼ ΔGBF

∴ `(DE)/(BF) = (GE)/(GB)`

`(DE)/30 = 8/16`

`\implies` DE = `30 xx 8/16` = 15 cm

c. In ΔABC and ΔDBE

DE || AC

∴ `(DB)/(AB) = (DE)/(AC)`

`(DB)/(AB) = 15/24 = 5/8`

`\implies` DB : AB = 5 : 8