Advertisements
Advertisements
प्रश्न
n = _______. This law is also called as Snell’s law.
पर्याय
`(sin "r")/(sin "i")`
`(sin "r")/(sin "e")`
`(sin "e")/(sin "r")`
`(sin "i")/(sin "r")`
उत्तर
n = `underline((sin "i")/(sin "r"))`. This law is also called as Snell’s law.
APPEARS IN
संबंधित प्रश्न
How refraction of light is related to refractive index?
The refractive indices of glass and water with respect to air are 3/2 and 4/3 respectively. If speed of light in glass is 2 x 108 m/s, find the speed of light in water.
A ray of light travelling in air enters obliquely into water. Does the light ray bend towards the normal or away from the normal? Why?
Find out, from Table, the medium having highest optical density. Also find the medium with lowest optical density.
| Material medium | Refractive index | Material medium | Refractive index |
| Air | 1.0003 | Canada Balsam | 1.53 |
| Ice | 1.31 | – | – |
| Water | 1.33 | Rock salt | 1.54 |
| Alcohol | 1.36 | – | – |
| Kerosene | 1.44 | Carbon disulphide | 1.63 |
| Fused quartz | 1.46 | Dense flint glass | 1.65 |
| Turpentine oil | 1.47 | Ruby | 1.71 |
| Benzene | 1.50 | Sapphire | 1.77 |
| Crown glass | 1.52 | Diamond | .42 |
The refractive index of diamond is 2.42. What is the meaning of this statement?
A ray of light travelling in water falls at right angles to the boundary of a parallel-sided glass block. The ray of light:
(a) is refracted towards the normal
(b) is refracted away from the normal
(c) does not get refracted
(d) is reflected along the same path
What is the unit of refractive index?
Which has higher refraction index : water of glass?
If the refractive index for light going from air to diamond be 2.42, what will be the refractive index from light going from diamond to air?
How is the refractive index of a material related to the speed of light in it?
Define Snell's law of refraction. A ray of light is incident on a glass slab at an angle of incidence of 60°. If the angle of refraction be 32.7°, calculate the refractive index of glass. (Given : sin 60° = 0.866, and sin 32.7° = 0.540).
The speed of light in vacuum and in two different glasses is given in the table below:
| Medium | Speed of light |
| Vacuum | 3.00 × 108 m/s |
| Flint glass | 1.86 × 108 m/s |
| Crown glass | 1.97 × 108 m/s |
(a) Calculate the absolute refractive indexes of flint glass and crown glass.
(b) Calculate the relative refractive index for light going from crown glass to flint glass.
The speed of light in water is 2.25 × 108 m/s. If the speed of light in vacuum be 3 × 108 m/s, calculate the refractive index of water.
Light enters from air into diamond which has a refractive index of 2.42. Calculate the speed of light in diamond. The speed of light in air is 3.0 × 108 ms−1.
State and explain the laws of refraction of light with the help of a labelled diagram.
The refractive indices of four materials A, B, C and D are 1.33, 1.43, 1.71 and 1.52 respectively. When the light rays pass from air into these materials, they refract the maximum in:
(a) material A
(b) material B
(c) material C
(d) material D
The refractive index of glass for light going from air to glass is The refractive index for light going from glass to air will be:
(a) `1/3`
(b) `4/5`
(c) `4/6`
(d) `5/2`
State the laws of refraction of light. Explain the term 'absolute refractive index of a medium' and write an expression to relate it with the speed of light in vacuum.
The refractive indices of water and glass with respect to air are 4/3 and 3/2 respectively. If the speed of light in glass is 2 × 108ms−1, find the speed of light in (i) air, (ii) water.
State Snell’s law of refraction of light. Write an expression to relate refractive index of a medium with speed of light in vacuum.
The refractive index of a medium ‘a’ with respect to medium ‘b’ is 2/3 and the refractive index of medium ‘b’ with respect to medium ‘c’ is 4/3. Find the refractive index of medium ‘c’ with respect to medium ‘a’.
State the law of refraction of light that defines the refractive index of a medium with respect to the other. Express it mathematically. How is refractive index of any medium 'A' with respect to a medium 'B' related to the speed of propagation of light in two media A and B? State the name of this constant when one medium is vacuum or air.
The refractive indices of glass and water with respect to vacuum are 3/2 and 4/3 respectively. If the speed of light in glass is 2 × 108m/s, find the speed of light in (i) vacuum, (ii) water.
Name a liquid whose mass density is less than that of water but it is optically denser than water.
Mark the correct answer in the question.
If the refractive index of glass with respect to air is 3/2, what is the refractive index of air with respect to glass?
If in a medium, the speed of light is 1.5 × 108 m/s how much will the absolute refractive index of that medium be?
The velocity of light in a medium is 1.5 x 188 m/s. What is the refractive index of the medium with respect to air, if the velocity in the air is 3 x 108 m/s?
Purple has the lowest refractive index.
If the absolute refractive index of water is 1.36, find the speed of light in water. (Speed of light in vacuum = 3 × 108 m/s.)
If the absolute refractive index of glass and water are 3/2 and 4/3 respectively, what is the refractive index of glass with respect to water?
Noor, a young student, was trying to demonstrate some properties of light in her Science project work. She kept ‘X’ inside the box (as shown in the figure) and with the help of a laser pointer made light rays pass through the holes on one side of the box. She had a small butter-paper screen to see the spots of light being cast as they emerged.

She measured the angles of incidence for both the rays on the left side of the box to be 48.60. She knew the refractive index of the material ‘X’ inside the box was 1.5. What will be the approximate value of angle of refraction?
(use the value: sin 48.6° ≈ 0.75)
The velocity of light in a vacuum is 3 x 108 ms-1 and in glass is 2 x 108 ms-1. The refractive index of glass is ______.
Match the Columns:
| Column ‘A’ | Column ‘B’ |
| Refractive index of water | (a) 1.31 |
| (b) 1.36 | |
| (c) 1.33 |
A light ray enters from medium A to medium B as shown in the figure.
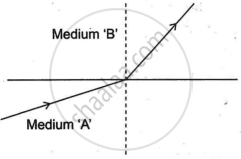
If the speed of light in medium A is `v_a` and in medium B is `v_b`, what is the refractive index of B with respect to A.
Absolute refractive indices of diamond and water are 2.42 and 1.33 respectively. Find the value of refractive index of water w.r.t. diamond.
| The ability of medium to refract light is expressed in terms of its optical density. Optical density has a definite connotation. It is not the same as mass density. On comparing two media, the one with the large refractive index is optically denser medium than the other. The other medium with a lower refractive index is optically rarer. Also the speed of light through a given medium is inversely proportional to its optical density. |
- Determine the speed of light in diamond if the refractive index of diamond with respect to vacuum is 2.42. Speed of light in vacuum is 3 × 108 m/s.
- Refractive indices of glass, water and carbon disulphide are 1.5, 1.33 and 1.62 respectively. If a ray of light is incident in these media at the same angle (say θ), then write the increasing order of the angle of refraction in these media.
- (A) The speed of light in glass is 2 × 108 m/s and is water is 2.25 × 108 m/s.
(a) Which one of the two optically denser and why?
(b) A ray of light is incident normally at the water glass interface when it enters a thick glass container filled with water. What will happen to the path of the ray after entering the glass? Give reason.
OR
(B) The absolute refractive indices of glass and water are 4/3 and 3/2, respectively. If the speed of light in glass is 2 × 108 m/s, calculate the speed of light in (i) vacuum (ii) water.
Absolute refractive indices of glass and water are `3/2 and 4/3` respectively. If the speed of light in glass is 2 × 108 m/s, the speed of light in water is ______.
