Advertisements
Advertisements
प्रश्न
Numerical problem.
Calculate the speed with which a satellite moves if it is at a height of 36,000 km from the Earth’s surface and has an orbital period of 24 hr (Take R = 6370 km) [Hint: Convert hr into seconds before doing calculation]
उत्तर
T = `"2π(R+h)"/"v"`
86400 =` "2 × 3.14 × (6370 + 36000)"/"v"`
v = 6.28 × 42370
266083.6 km/sec
S = `"d"/"t"`
= `266083/24`
= 11086.79 km/h
APPEARS IN
संबंधित प्रश्न
If the height of a satellite completing one revolution around the earth in T seconds is h1 meter, then what would be the height of a satellite taking \[2\sqrt{2} T\] seconds for one revolution?
Mahendra and Virat are sitting at a distance of 1 metre from each other. Their masses are 75 kg and 80 kg respectively. What is the gravitational force between them? G = 6.67 x 10-11 Nm2/kg2
A group of students from COEP Pune sent a small satellite _______ through ISRO in 2016.
What is Medium Earth Orbit?
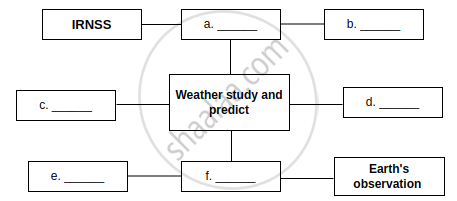
Write functions of Military satellite and Navigational satellite.
Note the relationship between the entries in all the three columns in the table and rewrite the table.
| Column-1 (Location) |
Column-2 Height from the earth’s surface (km) |
Column-3 g (m/s2) |
| Earth’s surface (average) | 8.8 | 0.225 |
| Mount Everest | 36.6 | 9.81 |
| Maximum height ever reached by manmade balloon | 400 | 9.8 |
| Orbit of a typical weather satellite | 35700 | 9.77 |
| Orbit of communication satellite | 0 | 8.7 |
Write a note on orbital velocity
How is a satellite maintained in nearly circular orbit?
Numerical problem.
At an orbital height of 400 km, find the orbital period of the satellite.
Complete the following equations: