Question

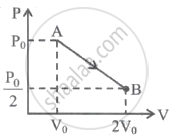
One mole of gas expands obeying the relation as shown in the P-V diagram. The maximum temperature in this process is equal to ______.

 

पर्याय

• `(P_0V_0)/R`

• `(3P_0V_0)/R`

• `(9P_0V_0)/(8R)`

• None of these

Answer 1

One mole of gas expands obeying the relation as shown in the P-V diagram. The maximum temperature in this process is equal to `underlinebb((9P_0V_0)/(8R)`.

Explanation:

The slope of the graph is, m = `P_0/(2V_0)`

The equation of this graph is given by,

P = `-(P_0V)/(2V_0) + (3P_0)/2`

= `(nRT)/V = -P_0/(2V_0) + (3P_0)/2`

⇒ `T - P_0/(2nRV_0)V^2 + (3P_0)/(2nR)V`

`(dT)/(dV) = -(2P_0V)/(2nRV_0) + (3P_0)/(2nR) = 0`

⇒ `(P_0V)/(nRV_0) = (3P_0)/(2nR)`

⇒ V = `3/2V_0`

At V = `(3V_0)/2`, the temperature will be maximum.

[n = 1]

`T_max = -P_0/(2RV_0) xx (9V_0^2)/4 + (3P_0)/(2R) xx (3V_0)/2`

⇒ `-9/8(P_0V_0)/R + 9/4 (P_0V_0)/R`

⇒ `9/8 (P_0V_0)/R`