Advertisements
Advertisements
प्रश्न
Out of 200 students; 35 students failed in MHT-CET, 40 in AIEEE and 40 in IIT entrance, 20 failed in MHT-CET and AIEEE, 17 in AIEEE and IIT entrance, 15 in MHT-CET and IIT entrance, and 5 failed in all three examinations. Find how many students : did not fail in any examination.
उत्तर
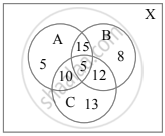
Let A = set of students who failed in MHT-CET
B = set of students who failed in AIEEE
C = set of students who failed in IIT entrance
X = set of all students
∴ n(X) = 200, n(A) = 35, n(B) = 40, n(C) = 40,
n(A ∩ B)= 20, n(B ∩ C) = 17, n(A ∩ C) = 15, n(A ∩ B ∩ C) = 5
n(A ∪ B ∪ C)
= n(A) + n(B) + n(C) – n(A ∩ B) – n(B ∩ C) – n(A ∩ C) + n(A ∩ B ∩ C)
= 35 + 40 + 40 - 20 - 17 – 15 + 5
= 68
∴ No. of students who did not fail in any exam
= n(X) – n(A ∪ B ∪ C)
= 200 – 68
= 132.
APPEARS IN
संबंधित प्रश्न
If n(A) = 15, n(A ∪ B) = 29, n(A ∩ B) = 7 then n(B) = ?
If A and B are subsets of the universal set X and n(X) = 50, n(A) = 35, n(B) = 20, n(A'∩ B') = 5, find: n(A ∪ B)
In a hostel, 25 students take tea, 20 students take coffee, 15 students take milk, 10 students take both tea and coffee, 8 students take both milk and coffee. None of them take tea and milk both and everyone takes at least one beverage, find the number of students in the hostel.
If A = {1,2,3} write the set of all possible subsets of A.
There are 260 persons with skin disorders. If 150 had been exposed to the chemical A, 74 to the chemical B, and 36 to both chemicals A and B, find the number of persons exposed to Chemical A or Chemical B.
If U = {x/x ∈ N, 1 ≤ x ≤ 12}, A = {1, 4, 7, 10}, B = {2, 4, 6, 7, 11}, C = {3, 5, 8, 9, 12} Write the set: A ∪ B.
If U = {x/x ∈ N, 1 ≤ x ≤ 12}, A = {1, 4, 7, 10}, B = {2, 4, 6, 7, 11}, C = {3, 5, 8, 9, 12} Write the set: B ∩ C.
If U = {x/x ∈ N, 1 ≤ x ≤ 12}, A = {1, 4, 7, 10}, B = {2, 4, 6, 7, 11}, C = {3, 5, 8, 9, 12} Write the set: A – B
If U = {x/x ∈ N, 1 ≤ x ≤ 12}, A = {1, 4, 7, 10}, B = {2, 4, 6, 7, 11}, C = {3, 5, 8, 9, 12} Write the set: B – C
If U = {x/x ∈ N, 1 ≤ x ≤ 12}, A = {1, 4, 7, 10}, B = {2, 4, 6, 7, 11}, C = {3, 5, 8, 9, 12} Write the set: A ∪ B ∪ C.
If U = {x/x ∈ N, 1 ≤ x ≤ 12}, A = {1, 4, 7, 10}, B = {2, 4, 6, 7, 11}, C = {3, 5, 8, 9, 12} Write the set: A ∩ (B ∪ C).
In a survey of 425 students in a school, it was found that 115 drink apple juice, 160 drink orange juice, and 80 drink both apple as well as orange juice. How many drinks neither apple juice nor orange juice?
Out of 200 students; 35 students failed in MHT-CET, 40 in AIEEE and 40 in IIT entrance, 20 failed in MHT-CET and AIEEE, 17 in AIEEE and IIT entrance, 15 in MHT-CET and IIT entrance, and 5 failed in all three examinations. Find how many students: failed in AIEEE or IIT entrance.
Out of all the patients in a hospital 89% are found to be suffering from heart ailment and 98% are suffering from lungs infection. If K% of them are suffering from both ailments, then K can not belong to the set ______.
