Advertisements
Advertisements
प्रश्न
Pressure decreases as one ascends the atmosphere. If the density of air is ρ, what is the change in pressure dp over a differential height dh?
उत्तर
Consider a horizontal parcel of air with cross-section A and height dh.
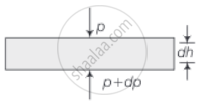
Let the pressure on the top surface and bottom surface be p and p + do. If the parcel is in equilibrium, then the net upward force must be balanced by the weight.
i.e., `(p + dp)A - pA = - pgAdh` ......(∵ Weight = Density × Volume × g)
= `- p xx Adh xx g`
⇒ `dp = - ρgdh` .....(ρ = density of air)
A negative sign shows that pressure decreases with height.
APPEARS IN
संबंधित प्रश्न
Does it matter if one uses gauge instead of absolute pressures in applying Bernoulli’s equation? Explain.
A barometer tube reads 76 cm of mercury. If the tube is gradually inclined keeping the open end immersed in the mercury reservoir, will the length of mercury column be 76 cm, more than 76 cm or less than 76 cm?
A barometer kept in an elevator accelerating upward reads 76 cm. The air pressure in the elevator is
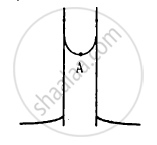
Figure shows a capillary tube of radius r dipped into water. If the atmospheric pressure is P0, the pressure at point A is
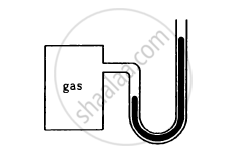
The heights of mercury surfaces in the two arms of the manometer shown in figure are 2 cm and 8 cm.
Atmospheric pressure = 1.01 × 105 N−2. Find (a) the pressure of the gas in the cylinder and (b) the pressure of mercury at the bottom of the U tube.
A closed vessel is half filled with water. There is a hole near the top of the vessel and air is pumped out from this hole.
(a) The water level will rise up in the vessel.
(b) The pressure at the surface of the water will decrease
(c) The force by the water on the bottom of the vessel will decrease
(d) The density of the liquid will decrease
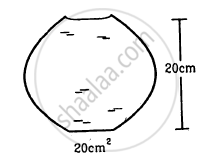
A glass full of water has a bottom of area 20 cm2, top of area 20 cm2, height 20 cm and volume half a litre.
(a) Find the force exerted by the water on the bottom.
(b) Considering the equilibrium of the water, find the resultant force exerted by the sides of the glass on the water. Atmospheric pressure = 1.0 × 105 N/m2. Density of water 1000 kg/m3 and g = 10 m/s2. Take all numbers
to be exact.
Suppose the glass of the previous problem is covered by a jar and the air inside the jar is completely pumped out. (a) What will be the answers to the problem? (b) Show that the answers do not change if a glass of different shape is used provided the height, the bottom area and the volume are unchanged.
If water be used to construct a barometer, what would be the height of water column at standard atmospheric pressure (76 cm of mercury) ?
A U-tube containing a liquid is accelerated horizontally with a constant acceleration a0. If the separation between the vertical limbs is l, find the difference in the heights of the liquid in the two arms.
