Advertisements
Advertisements
प्रश्न
Red queens and black jacks are removed from a pack of 52 playing cards. A card is drawn at random from the remaining cards, after reshuffling them. Find the probability that the card drawn is a face card
उत्तर
In a pack of 52 playing cards, there are 2 red queens and 2 black jacks.
Red queens and black jacks are removed from the pack. Then,
Number of remaining cards = 52 − 4 = 48
∴ Total number of outcomes = 48
There are 12 face cards in the pack, out of which 2 red queens and 2 black jacks are removed.
Number of face cards in the remaining pack of cards = 12 − (2 + 2) = 8
So, the favourable number of outcomes are 8.
∴ P(drawing a face card) =
APPEARS IN
संबंधित प्रश्न
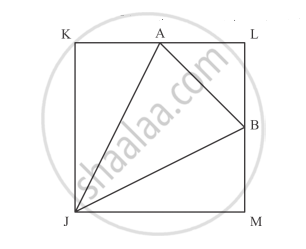
In the Fig. below, JKLM is a square with sides of length 6 units. Points A and B are the
mid- points of sides KL and LM respectively. If a point is selected at random from the
interior of the square. What is the probability that the point will be chosen from the interior of ΔJAB?
All the red face cards are removed from a pack of 52 playing cards. A card is drawn at random from the remaining cards, after reshuffling them. Find the probability that the drawn card is an ace.
In fig. 7 is shown a disc on which a player spins an arrow twice. The fraction `a/b` is formed, where 'a' is the number of sector on which arrow stops on the first spin and 'b' is the number of the sector in which the arrow stops on second spin. On each spin, each sector has equal chance of selection by the arrow. Find the probability that the fraction `a/b>1.`

A bag contains 3 red balls and 5 black balls. A ball is draw at random from the bag. What is the probability that the ball drawn is red?
A box contains 5 red marbels, 8 white marbles and 4 green marbles, One marble is taken out of the box at ramdom. What is the probability that the marble taken out will be not green?
The king, queen and jack of clubs are removed form a deck of 52 playing cards and the remaining cards are shuffled. A card is drawn from the remaining cards. Find the probability of getting a card of a queen of diamond.
In a bag there are 44 identical cards with figure of circle or square on them. There are 24 circles, of which 9 are blue and rest are green and 20 squares of which 11 are blue and rest are green. One card is drawn from the bag at random. Find the probability that it has the figure of blue circle and
A number is chosen at random from the number –3, –2, –1, 0, 1, 2, 3. What will be the probability that square of this number is less then or equal to 1?
In the probability that the digit is a multiple of 3 is
Two different coins are tossed simultaneously. The probability of getting at least one head is
It is known that a box of 200 electric bulbs contains 16 defective bulbs. One bulb is taken out at random from the box. What is the probability that the bulb drawn is non-defective.
Basketball players John, Vasim, Akash were practising the ball drop in the basket. The probabilities of success for John, Vasim and Akash are `4/5`, 0.83 and 58% respectively. Who had the greatest probability of success?
A die is thrown once. Find the probability of getting a number which is a prime number
A bag contains a black ball, a red ball and a green ball, all the balls are identical in shape and size. A ball is drawn from the bag without looking into it. What is the probability that the ball drawn is:
(i) a red ball
(ii) not a red ball
(iii) a white ball.
A bag contains 3 white, 5 black, and 2 red balls, all of the same shape and size. A ball is drawn from the bag without looking into it, find the probability that the ball drawn is not a red ball.
In a single throw of a die, find the probability of getting :
(i) 8
(ii) a number greater than 8
(iii) a number less than 8
From 10 identical cards, numbered 1, 2, 3, ……, 10, one card is drawn at random. Find the probability that the number on the card drawn is a multiple of
2
If the probability of an event is p, the probability of its complementary event will be ______.
The total number of events of throwing 10 coins simultaneously is ____________.
A lot consists of 144 ball pens of which 20 are defective and the others are good. Tanu will buy a pen if it is good but will not buy it if it is defective. The shopkeeper draws one pen at random and gives it to her. The probability that she will buy that pen is ____________.
