Question

Resolve the following rational expressions into partial fractions

`(x^2 + x + 1)/(x^2 - 5x + 6)`

Answer 1

`(x^2 + x + 1)/(x^2 - 5x + 6)`

Here the degree of the numerator is equal to the degree of the denominator.

Let us divide the numerator by the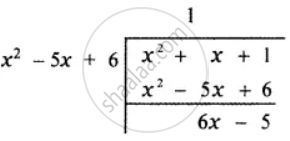

∴ `(x^2 + x + 1)/(x^2 - 5x + 6) = 1 + (6x - 5)/(x^2 - 5x + 6)`   ......(1)

Consider `(6x - 5)/(x^2 - 5x + 6)`

`(6x - 5)/(x^2 - 5x + 6) = (6x - 5)/(x^2 - 3x - 2x + 6)`

`(6x - 5)/(x^2 - 5x + 6) = (6x - 5)/(x(x - 3) - 2(x - 3))`

`(6x - 5)/(x^2 - 5x + 6) = (6x - 5)/((x - 2)(x - 3))`

`(6x - 5)/(x^2 - 5x + 6) = "A"/(x - 2) + "B"/(x - 3)`  ......(2)

`(6x - 5)/(x^2 - 5x + 6) = ("A"(x - 3) + "B"(x - 2))/((x - 2)(x - 3))`

6x – 5 = A(x – 3) + B(x – 2)   ......(3)

Put x = 3 in equation (3)

6(3) – 5 = A(3 – 3) + B(3 – 2)

18 – 5 = 0 + B

⇒ B = 13

Put x = 2 in equation (3)

6(2) – 5 = A(2 – 3) + B(2 – 2)

12 – 5 = – A + 0

7 = – A

⇒ A = – 7

Substituting the values of A and B in equation (2)

We have `(6x - 5)/(x^2 - 5x + 6) = (-7)/(x - 2) + 13/(x - 3)`

∴ The required partial fractions is

`(x^2 + x + 1)/(x^2 - 5x + 6) = 1 - 7/(x - 2) + 13/(x - 3)`