Advertisements
Advertisements
प्रश्न
Solve the following system of linear equations graphically:
x + 2y = 3, 2x − 3y + 8 = 0
उत्तर
Our equations are
x + 2y = 3 ....(1)
2x − 3y = −8 ...(2)
For Equation (1)
x + 2y = 3
Putting x = 0
0 + 2y = 3
2y = 3
y = `3/2`
y = 1.5
So, x = 0, y = 1.5 is a solution
i.e. (0, 1.5), is a solution.
x + 2y = 3
Putting y = 0
x + 2(0) = 3
x + 0 = 3
x = 3
So, x = 3, y = 0 is a solution.
x + 2y = 3
| x | 0 | 3 |
| y | 1.5 | 0 |
For Equation (2)
2x − 3y = −8
Putting x = 0
2(0) − 3y = −8
−3y = −8
Y = `(−8)/(−3)`
y = 2.67
So, x = 0, y = 2.67 is a solution
i.e. (0, 2.67) is a solution
2x − 3y = 8
Putting y = 0
2x − 3(0) = −8
2x = −8
x = `(−8)/2`
x = −4
So, x = −4, y = 0 is a solution
i.e., (−4, 0) is a solution
| x | 0 | −4 |
| y | 2.67 | 0 |
we will plot both equations on the graph
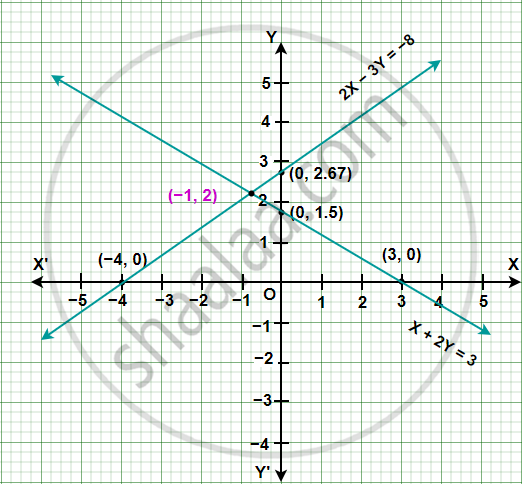
Since lines intersect at (−1, 2)
∴ x = −1, y = 2 is the solution of the pair of equations.
