Advertisements
Advertisements
प्रश्न
State which of the following pairs of triangles are congruent. If yes, write them in symbolic form (you may draw a rough figure).
∆ABC: AB = 4.8 cm, ∠A = 90°, AC = 6.8 cm
∆XYZ: YZ = 6.8 cm, ∠X = 90°, ZX = 4.8 cm
उत्तर

Both triangles are not congruent.
APPEARS IN
संबंधित प्रश्न
Given below are measurements of some parts of two triangles. Examine whether the two triangles are congruent or not, by using the SAS congruence rule. If the triangles are congruent, write them in symbolic form.
∆ABC, AB = 7 cm, BC = 5 cm, ∠B = 50°.
∆DEF, DE = 5 cm, EF = 7 cm, ∠E = 50°.
Given below are measurements of some parts of two triangles. Examine whether the two triangles are congruent or not, by using the SAS congruence rule. If the triangles are congruent, write them in symbolic form.
∆ABC, AB = 4.5 cm, AC = 4 cm, ∠A = 60°.
∆DEF, DE = 4 cm, FD = 4.5 cm, ∠D = 55°.
In Fig,
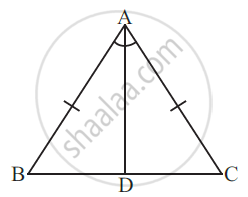
AB = AC and AD is the bisector of ∠BAC.
(i) State three pairs of equal parts in triangles ADB and ADC.
(ii) Is ∆ADB ≅ ∆ADC? Give reasons.
(iii) Is ∠B = ∠C? Give reasons.
Construct a triangle PQR with given conditions.
∠P = 60°, ∠R = 35° and PR = 7.8 cm
Construct a triangle PQR with given conditions.
∠Q = 90°, ∠R = 42° and QR = 5.5 cm
In ∆ABC and ∆PQR, ∠A = 50° = ∠P, PQ = AB, and PR = AC. By which property ∆ABC and ∆PQR are congruent?
In the given figure, which pairs of triangles are congruent by SAS congruence criterion (condition)? if congruent, write the congruence of the two triangles in symbolic form.

In the given figure, which pairs of triangles are congruent by SAS congruence criterion (condition)? if congruent, write the congruence of the two triangles in symbolic form.
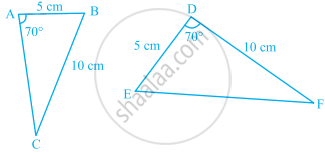
In the given figure, which pairs of triangles are congruent by SAS congruence criterion (condition)? if congruent, write the congruence of the two triangles in symbolic form.
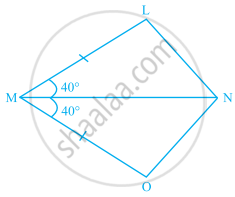
State which of the following pairs of triangles are congruent. If yes, write them in symbolic form (you may draw a rough figure).
∆PQR: PQ = 3.5 cm, QR = 4.0 cm, ∠Q = 60°
∆STU: ST = 3.5 cm, TU = 4 cm, ∠T = 60°
