Advertisements
Advertisements
प्रश्न
Sum of the first 14 terms of and AP is 1505 and its first term is 10. Find its 25th term.
उत्तर
Let d be the common difference of the AP.
Here, a = 10 and n = 14
Now,
S14 = 1505 (Given)
`⇒ 14/2 [2 xx 10 + (14 - 1) xx d ] = 1505 { S_n = n/2 [ 2a +(n-1) d]}`
⇒ 7(20 +13d)= 1505
⇒ 20+13d = 215
⇒ 13d = 215 -20 = 195
⇒ d= 15
∴ 25th term of the AP, a25
= 10 + (25-1) × 15 [ an = a+(n-1) d]
= 10+360
=370
Hence, the required term is 370.
APPEARS IN
संबंधित प्रश्न
The sum of the first 7 terms of an AP is 182. If its 4th and 17th terms are in the ratio 1:5, find the AP.
The sum of the first 9 terms of an AP is 81 and that of its first 20 terms is 400. Find the first term and common difference of the AP.
An AP 5, 12, 19, .... has 50 term. Find its last term. Hence, find the sum of its last 15 terms.
An AP 8, 10, 12, … has 60 terms. Find its last term. Hence, find the sum of its last 10 terms.
The sum of first q terms of an AP is (63q – 3q2). If its pth term is –60, find the value of p. Also, find the 11th term of its AP.
In a school, students decided to plant trees in and around the school to reduce air pollution. It was decided that the number of trees that each section of each class will plant will be double of the class in which they are studying. If there are 1 to 12 classes in the school and each class has two section, find how many trees were planted by student. Which value is shown in the question?
Which of the following sequences are A.P.? If they are A.P. find the common difference.
`3, 3 + sqrt2, 3 + 2sqrt2, 3 + 3sqrt2, ...`
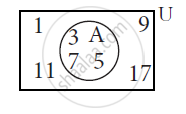
Observe the adjacent Venn diagram and write the complement of A.
Write a trinomial of degree 7.
In the A.P. 2, –2, –6, –10, ..... common difference (d) is ______.
