Question

The angle of elevation of an aircraft from a point A on the ground is 60°. After a flight of 30 seconds, the angle of elevation changes to 30°. The aircraft is flying at a constant height of 3500`sqrt3` m at a uniform speed. Find the speed of the aircraft.

Answer 1

Let the speed of an aircraft be x m/s.

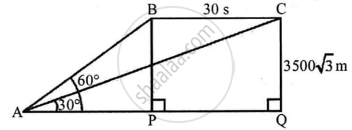
Distance = Speed × time = x × 30 

BC= 30x m

In ΔAPB, tan A = `(BP)/(AP)`

tan 60° = `(3500sqrt3)/(AP) = sqrt3`

∴ AP = 3500 m

In ΔACQ tan A = `(CQ)/(AQ)`

tan 30° = `(3500sqrt3)/(3500 + 30x)`

`1/sqrt3 = (3500sqrt3)/(3500 + 30x)`

10500 = 3500 + 30x

10500 − 3500 = 7000 + 30x

∴ x = `7000/30 = 700/3`

Speed of aircraft = `700/30` m/s

= `700/3 xx 18/5` km/h

= 140 × 6 km/h

∴ Speed aircraft = 840 km/h