Question

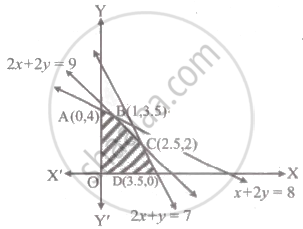
The point which provides the solution to the linear programming problem: Max P = 2x + 3y subject to constraints: x ≥ 0, y ≥ 0, 2x + 2y ≤ 9, 2x + y ≤ 7, x + 2y ≤ 8, is ______ 

पर्याय

• (3, 2.5)

• (2, 3.5)

• (2, 2.5)

• (1, 3.5)

Answer 1

The point which provides the solution to the linear programming problem: Max P = 2x + 3y subject to constraints: x ≥ 0, y ≥ 0, 2x + 2y ≤ 9, 2x + y ≤ 7, x + 2y ≤ 8, is (1, 3.5).

Explanation:

Feasible region lies on the origin side of all lines and in the first quadrant.

The comer points of the feasible region are 

O(0, 0), A(0, 4), B`(1, 7/2)`, C`(5/2, 2)`, D`(7/2, 0)`

Substituting the above points in P = 2x + 3y, we get

Max P = 12.5 at B `(1, 7/2)`

∴ B ≡ (1, 3.5)