Advertisements
Advertisements
प्रश्न
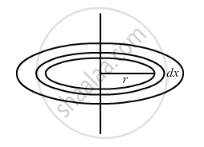
The surface density (mass/area) of a circular disc of radius a depends on the distance from the centre as [rholeft( r right) = A + Br.] Find its moment of inertia about the line perpendicular to the plane of the disc thorough its centre.
उत्तर
Consider a ring of thickness dx at a distance r from the centre of the disc.
Mass of the ring,
\[dm = \left( A + Br \right) \times 2\pi r \times dr\]
Therefore, moment of inertia about the centre,
\[I = \int_0^a r^2 dm\]
\[= \int_o^a d\left( A + Br \right) 2\pi r . dr \times r^2 \]
\[ = \int_o^a 2\pi A r^3 dr + \int_o^a 2\pi B r^4 dr\]
\[ = \left[ 2\pi A \left( \frac{r^4}{4} \right) + 2\pi B \left( \frac{r^5}{5} \right) \right]_0^a \]
\[ = 2\pi\left( \frac{A a^4}{4} + \frac{B a^5}{5} \right)\]
APPEARS IN
संबंधित प्रश्न
If the ice at the poles melts and flows towards the equator, how will it affect the duration of day-night?
A hollow sphere, a solid sphere, a disc and a ring all having same mass and radius are rolled down on an inclined plane. If no slipping takes place, which one will take the smallest time to cover a given length?
A circular disc A of radius r is made from an iron plate of thickness t and another circular disc B of radius 4r is made from an iron plate of thickness t/4. The relation between the moments of inertia IA and IB is __________ .
A cubical block of mass M and edge a slides down a rough inclined plane of inclination θ with a uniform velocity. The torque of the normal force on the block about its centre has a magnitude
A thin circular ring of mass M and radius r is rotating about its axis with an angular speed ω. Two particles having mass m each are now attached at diametrically opposite points. The angular speed of the ring will become
The centre of a wheel rolling on a plane surface moves with a speed \[\nu_0\] A particle on the rim of the wheel at the same level as the centre will be moving at speed ___________ .
A solid sphere, a hollow sphere and a disc, all having same mass and radius, are placed at the top of a smooth incline and released. Least time will be taken in reaching the bottom by _________ .
In the previous question, the smallest kinetic energy at
the bottom of the incline will be achieved by ___________ .
Three particles, each of mass 200 g, are kept at the corners of an equilateral triangle of side 10 cm. Find the moment of inertial of the system about an axis joining two of the particles.

Three particles, each of mass 200 g, are kept at the corners of an equilateral triangle of side 10 cm. Find the moment of inertial of the system about an axis passing through one of the particles and perpendicular to the plane of the particles.

Find the moment of inertia of a pair of spheres, each having a mass mass m and radius r, kept in contact about the tangent passing through the point of contact.
The radius of gyration of a uniform disc about a line perpendicular to the disc equals its radius. Find the distance of the line from the centre.
Find the moment of inertia of a uniform square plate of mass m and edge a about one of its diagonals.
Because of the friction between the water in oceans with the earth's surface the rotational kinetic energy of the earth is continuously decreasing. If the earth's angular speed decreases by 0⋅0016 rad/day in 100 years find the average torque of the friction on the earth. Radius of the earth is 6400 km and its mass is 6⋅0 × 1024 kg.
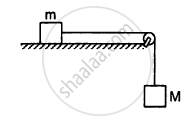
The following figure shows two blocks of mass m and M connected by a string passing over a pulley. The horizontal table over which the mass m slides is smooth. The pulley has a radius r and moment of inertia I about its axis and it can freely rotate about this axis. Find the acceleration of the mass M assuming that the string does not slip on the pulley.
A metre stick weighing 240 g is pivoted at its upper end in such a way that it can freely rotate in a vertical place through this end (see the following figure). A particle of mass 100 g is attached to the upper end of the stick through a light string of length 1 m. Initially, the rod is kept vertical and the string horizontal when the system is released from rest. The particle collides with the lower end of the stick and sticks there. Find the maximum angle through which the stick will rise.