Advertisements
Advertisements
प्रश्न
Three cubes of iron whose edges are 6 cm, 8 cm and 10 cm, respectively are melted and formed into a single cube. Find the edge of the new cube formed.
उत्तर
We have,
Edges of the cubes : a1 = 6 cm, a2 = 8 cm and a3 = 10 cm
Let the edge of the new cube so formed `= a_1^3 + a_2^3 + a_3^3`
⇒ a3 = 63 + 83 + 103
⇒ a3 = 216 + 512 + 1000
⇒ a3 = 1728
`⇒ a = root(3)(1728)`
∴ a = 12 cm
So, the edge of the new cube so formed is 12 cm.
APPEARS IN
संबंधित प्रश्न
An iron pillar consists of a cylindrical portion 2.8 m high and 20 cm in diameter and a cone 42 cm high is surmounting it. Find the weight of the pillar, given that 1 cubic cm of iron weighs 7.5 gm.
A sphere and a cube have equal surface areas. What is the ratio of the volume of the sphere to that of the cube?
The curved surface area of a cylinder is 264 m2 and its volume is 924 m3. The ratio of its diameter to its height is
A farmer connects a pipe of internal diameter 25 cm from a canal into a cylindrical tank in his field, which is 12 m in diameter and 2.5 m deep. If water flows through the pipe at the rate of 3.6 km/hr, then in how much time will the tank be filled? Also, find the cost of water if the canal department charges at the rate of ₹ 0.07 per m3.
Choose the correct answer of the following question:
The radii of the circular ends of a bucket of height 40 cm are 24 cm and 15 cm. The slant height (in cm) of the bucket is
The ratio between the volume of two spheres is 8 : 27. What is the ratio between their surface areas?
A toy is in the form of a cone mounted on a hemisphere of common base radius 7 cm. The total height of the toy is 31 cm. Find the total surface area of the toy.
The capacity of a cylindrical vessel with a hemispherical portion raised upward at the bottom as shown in the figure is `(pir^2)/3 [3h - 2r]`.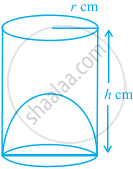
Two identical cubes each of volume 64 cm3 are joined together end to end. What is the surface area of the resulting cuboid?
A cylindrical tank has a radius of 154 cm. It is filled with water to a height of 3 m. If water to a height of 4.5 m is poured into it, what will be the increase in the volume of water in kl?
