Advertisements
Advertisements
प्रश्न
Two parallel S.H.M.s represented by `"x"_1 = 5 sin(4π"t" + π//3)` cm and `"x"_2 = 3sin (4π"t" + π//4)` cm are superposed on a particle. Determine the amplitude and epoch of the resultant S.H.M.
उत्तर
Given:
Two parallel S.H.Ms represented by `"x"_1 = 5 sin(4π"t" + π//3)` cm and `"x"_2 = 3 sin(4π"t" + π//4)` cm are superposed on a particle.
To find: The amplitude and epoch of the resultant SHM.
using superposition principle,
amplitude of resultant is given by,
A = `sqrt("A"_1^2 + "A"_2^2 + 2"A"_1"A"_2 "cos" phi)`
here, A₁ = 5, A₂ = 3 and Φ = `π/3 - π/4 = π/12`
now A = `sqrt(5^2 + 3^2 + 2(5)(3)cos(π/12))`
`= sqrt(25 + 9 + 30 xx 0.966)`
`= sqrt(62.98)`
= 7.936 cm
epoch of the resultant, θ = tan¯¹ `[("A"_1 "sin" Φ_1 + "A"_2 "sin" Φ_2)/("A"_1 "cos" Φ_1 + "A"_2 cos Φ_2)]`
= tan¯¹ `[(5sin(π/3) + 3sin(π/4))/(5cos(π/3) + 3cos(π/4))]`
= tan¯¹`[(5 × sqrt3/2 + 3 × 1/sqrt2)/(5 × 1/2 + 3 × 1/sqrt2)]`
= tan¯¹ `[(5 sqrt3 + 3 sqrt2)/(5 + 3sqrt2)]`
= 54° 23'
Therefore, the amplitude is 7.936 cm, and the epoch of the resultant is 54°23'.
APPEARS IN
संबंधित प्रश्न
Show that a linear S.H.M. is the projection of a U.C.M. along any of its diameter.
What does the phase of π/2 indicate in linear S.H.M.?
Define linear S.H.M.
The equation of a simple harmonic motion is given by, x = 8 sin (8πt) + 6 cos (8πt), the initial phase angle is ______
At extreme positions of a particle executing simple harmonic motion, ______
In a spring-block system, length of the spring is increased by 5%. The time period will ____________.
A particle is performing a linear simple harmonic motion of amplitude 'A'. When it is midway between its mean and extreme position, the magnitudes of its velocity and acceleration are equal. What is the periodic time of the motion?
A particle executes simple harmonic motion and is located at x = a, b, and c at times t0, 2t0, and 3t0 respectively. The frequency of the oscillation is ______.
A particle is executing simple harmonic motion with frequency f. The frequency at which its kinetic energy changes into potential energy is ______.
The velocities of a particle performing linear S.H.M are 0.13 m/s and 0.12 m/s, when it is at 0.12 m and 0.13 m from the mean position respectively. If the body starts from mean position, the equation of motion is ____________.
A particle executes simple harmonic motion with amplitude 'A' and period 'T'. If it is halfway between mean position and extreme position, then its speed at that point is ______.
The equation of a particle executing simple harmonic motion is given by x = sin π `("t" + 1/3)` m. At t = 1s, the speed of particle will be ______. (Given π = 3.14)
A particle is executing simple harmonic motion with amplitude A. When the ratio of its kinetic energy to the potential energy is `1/4`, its displacement from its mean position is ______.
For a particle executing SHM the displacement x is given by x = A cos ωt. Identify the graph which represents the variation of potential energy (P.E.) as a function of time t and displacement x.


A light rod of length 2m suspended from the ceiling horizontally by means of two vertical wires of equal length. A weight W is hung from a light rod as shown in figure.
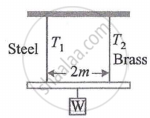
The rod hung by means of a steel wire of cross-sectional area A1 = 0.1 cm2 and brass wire of cross-sectional area A2 = 0.2 cm2. To have equal stress in both wires, T1/T2 = ______.
For a particle performing linear S.H.M., its average speed over one oscillation is ______. (a = amplitude of S.H.M., n = frequency of oscillation)
If a body is executing simple harmonic motion, then ______.
Two simple harmonic motion are represented by the equations, y1 = 10 sin `(3pi"t"+pi/4)` and y2 = 5`(3sin3pi"t"+sqrt3cos3pi"t")`. Their amplitudes are in the ratio of ______.
What do you know about restoring force?
The displacement of a particle performing simple harmonic motion is `1/3` rd of its amplitude. What fraction of total energy will be its kinetic energy?
