Advertisements
Advertisements
प्रश्न
Use a graph paper to answer the following questions (Take 1 cm = 1 unit on both axes)
1) Plot A(4,4), B(4,-6) and C(8,0), the vertices of a triangle ABC.
2) Reflect ABC on the y-axis and name it A’B’C’.
3) Write the coordinates of the images A’, B’ and C’.
4) Give a geometrical name for the figure AA’ C’ B’ BC.
5) Identify the line of symmetry of AA’ C’ BC’.
उत्तर
1) The points A(4, 4), B(4, -6) and C(8, 0) are plotted on the coordinate plane as follows:

2) To reflect the points ABC across Y axis we will keep y coordinate as it is and negate the x coordinate.

The reflected image of the triangle is shown in blue.
3) The coordinates of point A’, B’, C’ are (-4, 4), (-4,-6) and (-8, 0) respectively.

5) The line of symmetry of AA’C’B’BC would be the y axis.
APPEARS IN
संबंधित प्रश्न
Use graph paper to answer the following questions. (Take 2 cm = 1 unit on both axes)
1) Plot the points A( -4, 2) and B(2, 4)
2) A' is the image of A when reflected at the y-axis. Plot it on the graph paper and write the coordinates of A'.
3) B' is the image of B when reflected on the line AA'. Write the coordinates of B'.
4) Write the geometric name of the figure ABA'B'.
5) Name a line of symmetry of the figure formed
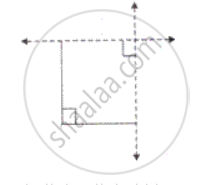
Complete the following figure, about the given lines of symmetry which are shown by dotted lines and name the figure thus obtained:
Draw a line of symmetry of semi-circle.
Which of the following alphabets has a right angle in it?
In figure, if B is the image of the point A with respect to the line l and P is any point lying on l, then the lengths of the line segments PA and PB are ______.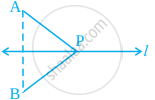
The digit having only one line of symmetry is ______.
With ruler and compasses, we can bisect any given line segment.
A circle has only 16 lines of symmetry.
Find the number of lines of symmetry for the following shape:

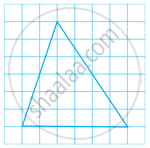
Copy the triangle in the following figure on squared paper. Draw the line(s) of symmetry, if any and identify the type of triangle. (Some of you may like to trace the figure and try paper-folding first!)