Advertisements
Advertisements
प्रश्न
Use graph paper for this question.
The point P (5, 3) was reflected in the origin to get the image P’.
(i) Write down the co-ordinates of P’.
(ii) If M is the foot of the perpendicular from of P to the X-axis, find the co-ordinates of M.
(iii) If N is the foot of the perpendicular from of P’ to the X-axis, find the co-ordinates of N.
(iv) Name the figure PMP’N.
(v) Find the area of die figure PMP’N.
उत्तर
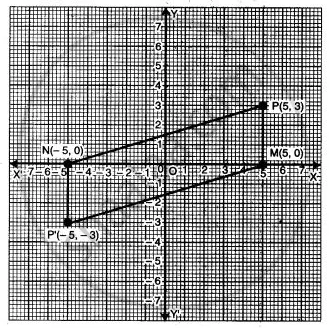
(i) P' (-5, -3).
(ii) M (5, 0).
(iii) N (-5, 0).
(iv) The figure PMP'N is a parallelogram.
(v) The area of figure PMP'N
= `(1)/(2) xx 10 xx 3 + (1)/(2) xx 10 xx 3`
= 15 + 15
= 30 sq. units.
APPEARS IN
संबंधित प्रश्न
State the co-ordinates of the following point under reflection in x-axis:
(3, 2)
State the co-ordinates of the following point under reflection in the line x = 0:
(–6, 4)
A point P is reflected in the origin. Co-ordinates of its image are (–2, 7). Find the co-ordinates of the image of P under reflection in the x-axis.
P' is the image of P under reflection in the x-axis. If the co-ordinates of P' are (2, 10), write the co-ordinates of P.
A point P is mapped onto P' under the reflection in the x-axis. P' is mapped onto P" under the reflection in the origin. If the co-ordinates of
P" are (5,-2), write down the co-ordinates of P. State the single transformation that takes place.
Find the co-ordinates of the image of A (-5, 4) after reflection in the line
y = 4
Write down the co-ordinates of the image of (5, – 4).
Reflection in y = 2.
Point A(4, – 1) is reflected as A’ on Y-axis. Point B on refletion on X-axis is mapped as B’ (- 2, 5).
(i) Write the co-ordinates of A’.
(ii) Write the co-ordinates of B.
(iii) Write the co-ordinates of the middle point
M of the segment A’B.
(iv) Write the co-ordinates of the point of reflection A” of A on X-axis.
The point P(3, 4) is reflected to P’ in the x-axis and O’ is the image of O (the Origin) in the line PP’ Find :
(i) The coordinates of P’ and O’.
(ii) The length of segment PP’ and OO’.
(iii) The perimeter of the quadrilateral POP’O’
(iv) What is the special name of the quadrilateral POP’O’.
