Advertisements
Advertisements
प्रश्न
Write the first five numbers in the third slanting row of the Pascal’s Triangle and find their squares. What do you infer?
उत्तर

Numbers in the 3rd standing row are 1, 3, 6, 10, 15, 21, ….
The squares are 12, 32, 62, 102, 152, 212, ….
= 1, 9, 36, 100, 225, 441, …
| Natural Number |
Cubes | Sum of the cubes | Squares of triangular Nos. |
| 1 | 13 = 1 | 1 | 1 |
| 2 | 23 = 8 | 1 + 8 = 9 | 9 |
| 3 | 23 = 27 | 1 + 8 + 27 + 36 | 36 |
| 4 | 43 = 64 | 1 + 8 + 27 + 64 = 100 | 100 |
| 5 | 53 = 125 | 1 + 8 + 27 + 64 + 125 = 225 | 225 |
| 6 | 63 = 216 | 1 + 8 + 27 + 64 + 125 + 216 = 441 | 441 |
| 7 | 73 = 343 | 1 + 8 + 27 + 64 + 125 + 216 + 343 = 784 | 784 |
| . | . | . | . |
| . | . | . | . |
| . | . | . | . |
From the above table we can conclude that the squares of the triangular numbers are the sum of cubes of natural numbers.
APPEARS IN
संबंधित प्रश्न
Complete the Pascal’s Triangle.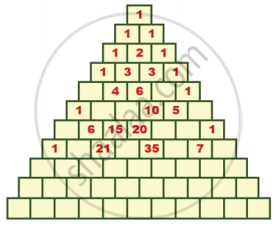
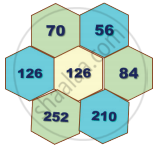
The following hexagonal shapes are taken from Pascal’s Triangle. Fill in the missing numbers.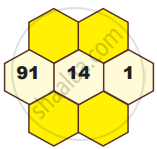
Complete the Pascal’s Triangle by taking the numbers 1, 2, 6, 20 as line of symmetry.
The difference between the consecutive terms of the fifth slanting row containing four elements of a Pascal’s Triangle is
Find the triangular numbers from the Pascal’s Triangle and colour them.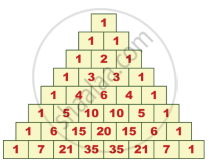
The following hexagonal shapes are taken from Pascal’s Triangle. Fill in the missing numbers.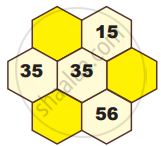
Verify whether the following hexagonal shapes form a part of the Pascal’s Triangle.
Verify whether the following hexagonal shapes form a part of the Pascal’s Triangle.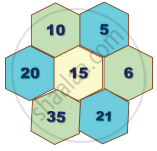
Verify whether the following hexagonal shapes form a part of the Pascal’s Triangle.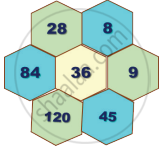
Verify whether the following hexagonal shapes form a part of the Pascal’s Triangle.