Advertisements
Advertisements
Question
A battery of e.m.f. 15 V and internal resistance 2 Ω is connected to two resistors of resistances 4 ohm and 6 ohm joined (a) in series. Find in each case the electrical energy spent per minute in 6 Ω resistor.
Solution
Given , E.m.f of battery , V = 15 V
Internal resistance of battery , RB = 2 Ω
Resistance given in circuit , R1 = 4 Ω
R2 = 6 Ω
(i) When resistors are connected in series ,
Equivalent resistance , R = RB + R1 + R2 = 12 Ω
Current in the circuit , I = `15/12` = 1.25 A
Now voltage across resistor R2 , V2 = IR = 1.25 × 6
V2 = 7.50 V
Time , t = 1 min = 60 sec
Energy across R2 , E = `("V"^2"t")/"R" = ((7.5)^2 xx 60)/6`
E = 562.5 J
APPEARS IN
RELATED QUESTIONS
What is the Flow of Charge Called?
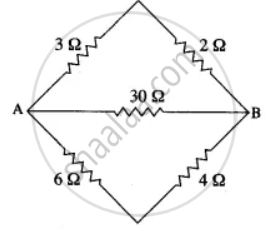
Calculate the equivalent resistance between A and B in the adjacent diagram.
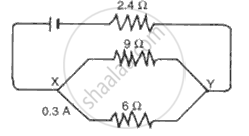
A current of 0.3 A is flowing through a branch of 6 Ω resistors in a junction as shown in fig. . Calculate the
(a) P.d. across the junction XY,
(b) Current flowing through 9Ω and 24Ω
( c) P.d. across 24 Ω resistor, and
( d) e.m.f. of the cell.
Kilowatt hour is the unit of
The power of an electric device is a product of ______ and ______.
Coulomb is the SI unit of ____________.
The number of electrons constituting a 1-coulomb charge is ______.
Assertion (A): Electric current will not flow between two charged bodies when connected if their charges are the same.
Reason (R): Current is the rate of flow of charge.
