Question

A block of mass m slides along a floor while a force of magnitude F is applied to it at an angle θ as shown in figure. The coefficient of kinetic friction is µK. Then, the block's acceleration 'a' is given by ______.

(g is acceleration due to gravity)

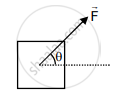
Options

• `"F"/"m"costheta-mu_"k"(g-"F"/"m"sintheta)`

• `"F"/"m"costheta-mu_"k"(g+"F"/"m"sintheta)`

• `"F"/"m"costheta+mu_"k"(g-"F"/"m"sintheta)`

• `-"F"/"m"costheta-mu_"k"(g-"F"/"m"sintheta)`

Answer 1

A block of mass m slides along a floor while a force of magnitude F is applied to it at an angle θ as shown in figure. The coefficient of kinetic friction is µK. Then, the block's acceleration 'a' is given by `bb("F"/"m"costheta-mu_"k"(g-"F"/"m"sintheta))`.

Explanation:

Block's free-body diagram,

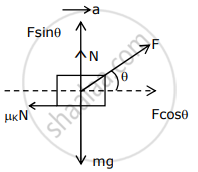
For equilibrium in the vertical direction,

F sinθ + N = mg

N = mg - F sinθ           ...(i)

Also, F cosθ - µ_kN = ma      ...(ii)

Solving (i) and (ii),

a = `"F"/"m"costheta -mu_k["g"-"F"/"m"sintheta]`