Question

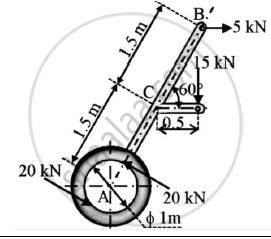
A machine part is subjected to forces as shown.Find the resultant of forces in magnitude and in direction.
Also locate the point where resultant cuts the centre line of bar AB.

Solution:
Given : A machine subjected to various forces
To find : Resultant of forces
             Point where the resultant force cuts the bar AB

Answer 1

 

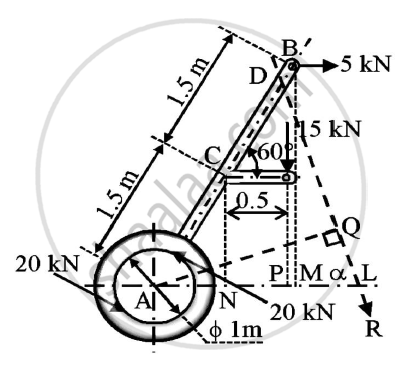
In △BAM,

∠A=60°
AB=3 m
BM=3sin60
      =2.5981 m

In △CAN
AC=1.5m
AN=1.5cos60
     =0.75 m
AP=AN+NP
    =0.75+0.5
    =1.25 m

Two 20N forces are equal and opposite in direction.Hence,they form a couple
Perpendicular distance between two 20 N forces = 1m
Moment of couple = 20 x 1
                               =20 kN-m (Anti clockwise)
Assume R is the resultant of the forces and it is inclined at an angle θ with horizontal

`ΣF_X=5 kN`

`ΣF_Y=-15 kN`
`R=sqrt( F_x^2)+ (F_Y^2)`
`=sqrt(5^2 +(−15)^2)`
=15.8114 kN

`θ = tan^(-1)((R_y)/(R_X))`
`=tan^(-1)((−15)/5)`

=71.5651° (in fourth quadrant)
Assume that the resultant cut the center line of bar AB at point D
Applying Varigon’s theorem

`ΣM_A=ΣM_A^R`
-5 x BM -15 x AP +20 = R x AQ
-11.7405 = -15.8114 x AQ
AQ = 0.7425 m

In △AQL, ∠ALQ=θ

∠QAL=90 - θ

∠BAL=60

∠QAD=60 - (90 - θ)

          = θ - 30
          =71.5651 - 30

           =41.5651°

In△DAQ,cos QAD= `(AQ)/(AD)`
`AD=(AQ)/(cosDAQ) = (0.7425)/(cos41.5651) = 0.9924 m`

Resultant force = 15.8114 kN(at 71.5651° in fourth quadrant)
It cuts the center line of bar AB at point D such that AD=0.9924m