Question

A rod of mass 'm' hinged at one end is free to rotate in a horizontal plane. A small bullet of mass m/4 travelling with speed 'u' hits the rod and attaches to it at its centre. Find the angular speed of rotation of rod just after the bullet hits the rod 3. [take length of the rod as 'l']

Options

• `6/19 "u"/"l"`

• `6/13 "u"/"l"`

• `3/19 "u"/"l"`

• `3/13 "u"/"l"`

Answer 1

`bb(6/19 "u"/"l")`

Explanation:

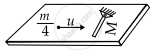
Bullet's angular momentum with respect to the pivot:

L_B = `"m"/4"u"("l"/2) = "mul"/8`

When the bullet strikes the rod, the system's angular momentum is:

L_s = [I_B + I_R] ω = `["m"/4("l"/2)^2+1/3"ml"^2]`ω 

= `19/48` ml²ω 

By conservation of angular momentum

`"mul"/8 = 19/48`ml²ω 

ω = `6/19"u"/"l"`