Question

A signal which can be green or red with probability 4/5 and 1/5 respectively, is received by station A and then trasmitted to station B. The probability of each station receiving the signal correctly is 3/4. If the signal received at station B is given, then the probability that the original signal is green, is

Options

• `3/5`

• `6/7`

• `20/23`

• `9/20`

Answer 1

`20/23`

Explanation:

From the tree diagram, it follows that

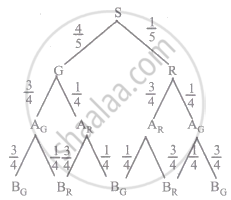
`P(B_G) = 46/80, P(G) = 4/5`

`P((B_G)/G) = 10/16 = 5/8`

∴ `P(B_G  ∩ G) = 5/8 xx 4/5 = 1/2`  ......`[(because P(B_G ∩ G)),(= P(B_G/G) xx P(G))]`

Now, `P(G/B_G) = (P(B_G ∩ G))/(P(B_G)) = 1/2 xx 80/40 = 20/23`