Advertisements
Advertisements
Question
A wheel rotating at a speed of 600 rpm (revolutions per minute) about its axis is brought to rest by applying a constant torque for 10 seconds. Find the angular deceleration and the angular velocity 5 seconds after the application of the torque.
Solution
Initial angular velocity of the wheel,
\[\omega_0 = 600 rpm\]
\[ = \frac{600}{60} = 10\text{ revolutions per second}\]
After 10 seconds,
Final angular velocity of the wheel,
\[\omega = 0\]
\[\text{So, }\omega_0 = - at\]
\[ \Rightarrow \alpha = - \frac{10}{10} = - 1 rev/ s^2 \]
Now, t = 5s
We know that
\[\omega' = \omega_0 + at\]
\[ \Rightarrow \omega' = 10 - 1 \times 5 = 5 rev/s\]
APPEARS IN
RELATED QUESTIONS
The moon rotates about the earth in such a way that only one hemisphere of the moon faces the earth (see the following figure). Can we ever see the "other face" of the moon from the earth? Can a person on the moon ever see all the faces of the earth?
A sphere rolls on a horizontal surface. If there any point of the sphere which has a vertical velocity?
A body is in pure rotation. The linear speed \[\nu\] of a particle, the distance r of the particle from the axis and the angular velocity \[\omega\] of the body are related as \[\omega = \frac{\nu}{r}.\] Thus
The following figure shows a small wheel fixed coaxially on a bigger one of double the radius. The system rotates about the common axis. The strings supporting A and B do not slip on the wheels. If x and y be the distance travelled by A and B in the same time interval, then _________ .

The angular velocity of the engine (and hence of the wheel) of a scooter is proportional to the petrol input per second. The scooter is moving on a frictionless road with uniform velocity. If the petrol input is increased by 10%, the linear velocity of the scooter is increased by ___________ .
Find the angular velocity of a body rotating with an acceleration of 2 rev/s2 as it completes the 5th revolution after the start.
A disc of radius 10 cm is rotating about its axis at an angular speed of 20 rad/s. Find the linear speed of the middle point of a radius.
A cylinder rotating at an angular speed of 50 rev/s is brought in contact with an identical stationary cylinder. Because of the kinetic friction, torques act on the two cylinders accelerating the stationary one and decelerating the moving one. If the common magnitude of the acceleration and deceleration be one revolution per second square, how long will it take before the two cylinders have equal angular speed?
A dumb-bell consists of two identical small balls of mass 1/2 kg each connected to the two ends of a 50 cm long light rod. The dumb-bell is rotating about a fixed axis thorough the centre of the rod and perpendicular to it at an angular speed of 10 rad/s. An impulsive force of average magnitude 5⋅0 N acts on one of the masses in the direction of its velocity for 0⋅10 s. Find the new angular velocity of the system.
A boy is standing on a platform which is free to rotate about its axis. The boy holds an open umbrella in his hands. The axis of the umbrella coincides with that of the platform. The moment of inertia of "the platform plus the boy system" is 3⋅0 × 10−3 kg-m2 and that of the umbrella is 2⋅0 × 10−3 kg-m2. The boy starts spinning the umbrella about the axis at an angular speed of 2⋅0 rev/s with respect to himself. Find the angular velocity imparted to the platform.
A ball falls on the ground from a height of 2.0 m and rebounds up to a height of 1.5 m. Find the coefficient of restitution.
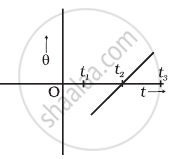
The variation of angular position θ, of a point on a rotating rigid body, with time t is shown in figure. Is the body rotating clock-wise or anti-clockwise?
Two cylindrical hollow drums of radii R and 2R, and of a common height h, are rotating with angular velocities ω(anti-clockwise) and ω(clockwise), respectively. Their axes, fixed are parallel and in a horizontal plane separated by (3R + δ). They are now brought in contact (δ → 0).
- Show the frictional forces just after contact.
- Identify forces and torques external to the system just after contact.
- What would be the ratio of final angular velocities when friction ceases?
