Advertisements
Advertisements
Question
Considering the pressure p to be proportional to the density, find the pressure p at a height h if the pressure on the surface of the earth is p0.
Advertisements
Solution
Let p0 be the density of air on the surface of the earth.
As per the question, pressure ∝ density
⇒ `p/p_0 = ρ/ρ_0`
⇒ `ρ = ρ_0/p_0 p`
∴ `dp = - (ρ_0g)/p_0 pdh` ......[∵ dp = – ρgdh]
⇒ `(dp)/p = - (ρ_0g)/p_0 dh`
⇒ `int_(p_0)^p (dp)/p = - (ρ_0 g)/p_0 int_0^h dh` .....`[(∵ at h = p, r = p_0),(and at h = h, p = p)]`
⇒ In `p/ρ_0 = - (ρ_0 g)/p_0 h`
By removing log, `p = p_0e (- (ρ_0gh)/p_0)`
APPEARS IN
RELATED QUESTIONS
Does it matter if one uses gauge instead of absolute pressures in applying Bernoulli’s equation? Explain.
During blood transfusion the needle is inserted in a vein where the gauge pressure is 2000 Pa. At what height must the blood container be placed so that blood may just enter the vein? [Use the density of whole blood from Table 10.1].
A one meter long glass tube is open at both ends. One end of the tube is dipped into a mercury cup, the tube is kept vertical and the air is pumped out of the tube by connecting the upper end to a suction pump. Can mercury be pulled up into the pump by this process?
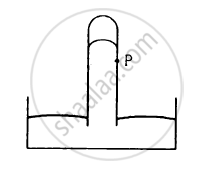
Consider the barometer shown in the following figure. If a small hole is made at a point P in the barometer tube, will the mercury come out from this hole?
The three vessels shown in the following figure have same base area. Equal volumes of a liquid are poured in the three vessels. The force on the base will be
A barometer kept in an elevator reads 76 cm when it is at rest. If the elevator goes up with increasing speed, the reading will be ______.
A barometer kept in an elevator accelerating upward reads 76 cm. The air pressure in the elevator is
Figure shows a capillary tube of radius r dipped into water. If the atmospheric pressure is P0, the pressure at point A is
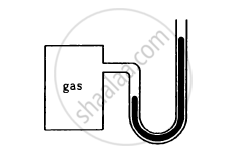
The heights of mercury surfaces in the two arms of the manometer shown in figure are 2 cm and 8 cm.
Atmospheric pressure = 1.01 × 105 N−2. Find (a) the pressure of the gas in the cylinder and (b) the pressure of mercury at the bottom of the U tube.
The area of cross section of the wider tube shown in figure is 900 cm2. If the boy standing on the piston weighs 45 kg, find the difference in the levels of water in the two tubes.
The weight of an empty balloon on a spring balance is W1. The weight becomes W2when the balloon is filled with air. Let the weight of the air itself be w. Neglect the thickness of the balloon when it is filled with air. Also neglect the difference in the densities of air inside and outside the balloon.
(a) W2 = W1
(b) W2 = W1 + w
(c) W2 < W1 + w
(d) W2 > W1
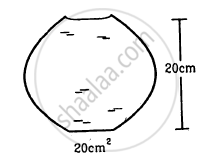
A glass full of water has a bottom of area 20 cm2, top of area 20 cm2, height 20 cm and volume half a litre.
(a) Find the force exerted by the water on the bottom.
(b) Considering the equilibrium of the water, find the resultant force exerted by the sides of the glass on the water. Atmospheric pressure = 1.0 × 105 N/m2. Density of water 1000 kg/m3 and g = 10 m/s2. Take all numbers
to be exact.
Suppose the glass of the previous problem is covered by a jar and the air inside the jar is completely pumped out. (a) What will be the answers to the problem? (b) Show that the answers do not change if a glass of different shape is used provided the height, the bottom area and the volume are unchanged.
Pressure decreases as one ascends the atmosphere. If the density of air is ρ, what is the change in pressure dp over a differential height dh?
A glass capillary sealed at the upper end is of length 0.11 m and internal diameter 2 × 10-5 m. This tube is immersed vertically into a liquid of surface tension 5.06 × 10-2 N/m. When the length x × 10-2 m of the tube is immersed in liquid then the liquid level inside and outside the capillary tube becomes the same, then the value of x is ______ m. (Assume atmospheric pressure is 1.01 × 105 `"N"/"m"^2`)
