Question

Construct an isosceles triangle using the given data: Altitude AD = 4cm and vertex ∠A = 90°

Answer 1

Altitude AD = 4cm and vertex ∠A = 90°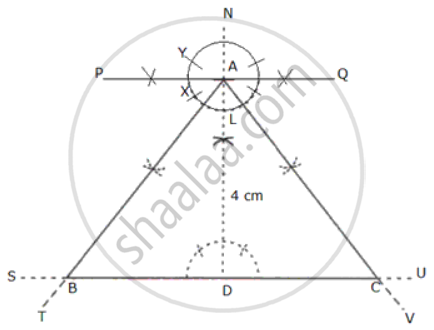

Steps of construction:
1. Draw a line a SU of any length.
2. Take a point D on SU.
3. Through the point D on SU draw ND perpendicular to SU.
4. With D as centre and radius 4cm, draw an arc to cut ND at A.
5. Construct ∠DAB = ∠DAC = `(1)/(2) xx 90°` = 45°.
(a) With A as centre, draw an arc cutting AD at L.
(b) With L as centre and same radius, cut the arc at X and Y.
(c) Using X and Y, draw PQ perpendicular to AD.
(d) Bisect ∠PAD and ∠QAD. Let AT and AV are the bisectors. AT and AV make an angle of 45° with AD.
(e) Mark the points as B and C where AR and AV meet SU.
Thus, ABC is the required triangle.