Question

Construct ΔLMN, in which ∠M = 60°, ∠N = 80° and LM + MN + NL = 11 cm.

Answer 1

Rough figure:

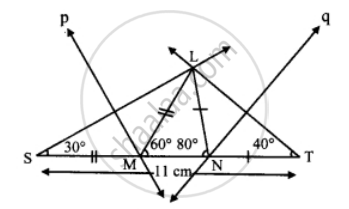
Explanation:

(i) As shown in the figure, take point S and T on line MN, such that

MS = LM and NT = LN     ...(i)

MS + MN + NT = ST      ...[S-M-N, M-N-T]

∴ LM + MN + LN = ST     ...(ii)

Also,

LM + MN + LN = 11 cm    …(iii)

∴ ST = 11 cm     ...[From (ii) and (iii)]

(ii) In ∆LSM

LM = MS

∴ ∠MLS = ∠MSL = x°     ...(iv) [isosceles triangle theorem]

In ∆LMS, ∠LMN is the exterior angle.

∴ ∠MLS + ∠MSL = ∠LMN      ...[Remote interior angles theorem]

∴ x + x = 60°      ...[From (iv)]

∴ 2x = 60°

∴ x = 30°

∴ ∠LSM = 30°

∴ ∠S = 30°

Similarly, ∠T = 40°

(iii) Now, in ∆LST

∠S = 30°, ∠T = 40° and ST = 11 cm

Hence, ALST can be drawn.

(iv) Since, LM = MS

∴ Point M lies on perpendicular bisector of seg LS.

Also LN = NT

∴ Point N lies on perpendicular bisector of seg LT.

∴ Points M and N can be located by drawing the perpendicular bisector of LS and LT respectively.

∴ ∆LMN can be drawn.

Steps of construction:​

1. Draw seg ST of length 11 cm.
2. From point S draw ray making angle of 30°.
3. From point T draw ray making angle of 40°.
4. Name the point of intersection of two rays as L.
5. Draw the perpendicular bisector of seg LS and seg LT intersecting seg ST in M and N respectively.
6. Join LM and LN.

Therefore, ∆LMN is the required triangle.