Advertisements
Advertisements
Question
DPQ is an isosceles triangle with DP = DQ. A straight line CD bisects the exterior ∠QDR. Prove that DC is parallel to PQ.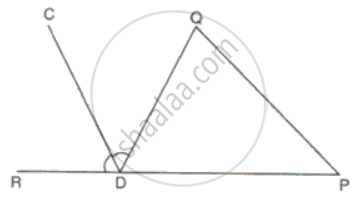
Sum
Solution
In ΔQDP,
DP = DQ
∴ ∠Q = ∠P
∠QDR = ∠Q + ∠P
2∠QDC = ∠Q + ∠P ....(DC bisects angle QDR)
2∠QDC = ∠Q + ∠Q = 2∠Q
∠QDC = ∠Q
But these are alternate angles.
∴ DC || PQ.
shaalaa.com
Is there an error in this question or solution?
