Question

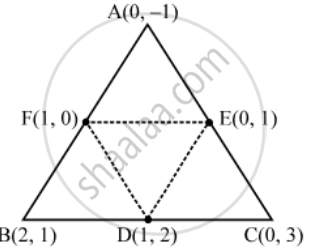
Find the area of the triangle formed by joining the mid-point of the sides of the triangle whose vertices are (0, –1), (2, 1) and (0, 3). Find the ratio of area of the triangle formed to the area of the given triangle.

Answer 1

Let A (0, –1), B(2, 1) and C(0, 3) be the vertices of ∆ABC.

Let D, E, F be the mid-points of sides BC, CA and AB respectively. Then, the coordinates of D, E and F are (1, 2), (0, 1) and (1, 0) respectively.

Now,

`⇒ D=((0+2)/2,(-1+1)/2)=(1,0) `

`⇒ E =((0+0)/2+(3-1)/2)=(0,1)`

`⇒ F = ((2+0)/2,(1+3)/2)=(1,2)`

Area of triangle=`1/2(x_1(y_2-y_3)+x_2(y_3-y_1)+x_3(y_1-y_2))`

Area of Δ DEF =`1/2(1(2-1)+1(1-0)+0(0-2))`

                       `=1 sq. units`

Area of Δ ABC =`1/2(0(1-3)+2{3-(-1)}+0(-1-1))`

                       `1/2{8}=4 sq. units`

∴ Area of ∆DEF : Area of ∆ABC = 1 : 4