Question

Find the area of the region bounded by the ellipse `x^2/4 + y^2/9` = 1.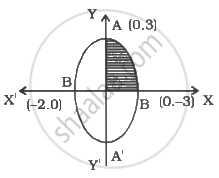

Options

• `pi` sq.units

• `2pi` sq.units

• `4pi` sq.units

• `6pi` sq.units

Answer 1

`6pi` sq.units

Explanation:

Given, `x^2/4 + y^2/9` = 1

⇒ `y^2/9 = 1 - x^2/4`

⇒ `y = 3/2 sqrt(4 - x^2)`

It is an ellipse centre (0, 0), the length of semi-major axis = 3 and that of semi-minor axis = 2

Area bounded by ellipse = 4 × Area of region AOB

= `4int_0^2 3/2 sqrt(4 - x^2)  dx`

= `6int_0^2  sqrt(4 - x^2)  dx`

= `6[(xsqrt(4 - x^2))/2 + 4/2  sin^-1  x/2]_0^2`

= `16[(0 + 2sin^-1) - (0 + sin^-1 (0))]`

= `6[2 - pi/2]`

= 6 = `6pi` sq.units.