Question

Find the asymptotes of the following curves:

f(x) = `(x^2 - 6x - 1)/(x + 3)`

Answer 1

No vertical asymptotes

Horizontal asymptotes RHL .......(Right Hand Limit)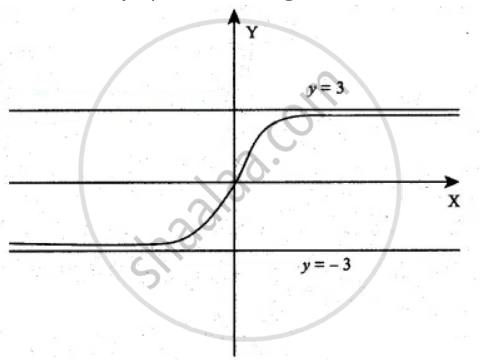

`lim_(x -> oo) (3x)/sqrt(x^2 + 2) = 3lim_(x -> oo) x/sqrt(x^2 + 2)`

= `3lim_(x -> oo) x/(x(sqrt(1 + 2)/x^2))`

= `3lim_(x -> oo) 1/sqrt((1 + 2/x^2)`

= `3 (lim_(x -> oo) (1))/(lim_(x -> oo) sqrt(1 + 2/x^2)`

= `3/(lim_(x -> oo) sqrt(1 + 2/x^2)`

= `3/1`

= 3

Similarly LHL  .....(Left Hand Limit) = – 3

i.e. `lim_(x -> oo) (- (3x)/sqrt(x^2 + 2)) = - 3 lim_(x -> oo) x/sqrt(x^2 + 2)` = – 3

y = 3 and y = – 3 are the Horizontal asymptotes

Slant asymptotes’.

No such slant asymptotes exist for the given curve.