Advertisements
Advertisements
Question
If ∆PQR and ∆XYZ are congruent under the correspondence QPR `leftrightarrow` XYZ, then QR = ______.
Solution
If ∆PQR and ∆XYZ are congruent under the correspondence QPR `leftrightarrow` XYZ, then QR = XZ.
APPEARS IN
RELATED QUESTIONS
Classify the given triangle based on its sides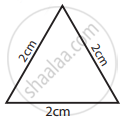
Classify the following triangle based on its sides and angles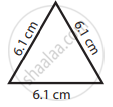
Can a triangle be formed with the following sides? If yes, name the type of triangle.
3.5 cm, 3.5 cm, 3.5 cm
The given triangle is _________
If for ∆ABC and ∆DEF, the correspondence CAB `leftrightarrow` EDF gives a congruence, then which of the following is not true?
If ∆PQR and ∆XYZ are congruent under the correspondence QPR `leftrightarrow` XYZ, then ∠P = ______.
If ∆PQR and ∆XYZ are congruent under the correspondence QPR `leftrightarrow` XYZ, then QP = ______.
If ∆PQR and ∆XYZ are congruent under the correspondence QPR `leftrightarrow` XYZ, then ∠Q = ______.
If ∆PQR and ∆XYZ are congruent under the correspondence QPR `leftrightarrow` XYZ, then RP = ______.
It is possible to have a triangle in which each angle is equal to 60°.
A one rupee coin is congruent to a five rupee coin.
If the areas of two squares is same, they are congruent.
If three angles of two triangles are equal, triangles are congruent.
If two sides and one angle of a triangle are equal to the two sides and angle of another triangle, then the two triangles are congruent.
