Question

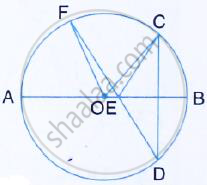
In the figure given below, AB is diameter of the circle whose centre is O. given that: ∠ECD =
∠EDC = 32°. Show that ∠COF = ∠CEF.

Answer 1

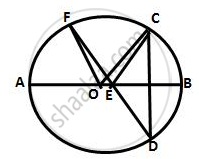
Here, ∠COF = 2 ∠CDF = 2 × 32° = 64°  ……… (i)
(Angle at the centre is double the angle at the circumference subtended by the same chord)
In ΔECD,
∠CEF = ∠ECD +∠EDC = 32° +32°  = 64°  ………….(ii)
(Exterior angle of a Δ is equal to the sum of pair of interior opposite angles)
From (i) and (ii), we get
∠COF =∠CEF