Question

In the given figure, triangle ABC is similar to triangle PQR. AM and PN are altitudes whereas AX and PY are medians.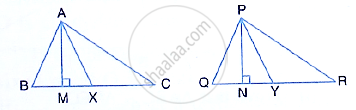
Prove that : `(AM)/(PN)=(AX)/(PY)`

Answer 1

Since ∆ABC ~ ∆PQR
So, their respective sides will be in proportion

0r `(AB)/(PQ)=(AC)/(PR)=(BC)/(QR)`
Also, ∠A = ∠P, ∠B = ∠Q, ∠C = ∠R
In ΔABM and ΔPQN,
∠ABM = ∠PQN (Since, ABC and PQR are similar)
∠AMB = ∠PNQ = 90°
∆ABM ~ ∆PQN(AA similarity)
`∴(AM)/(PN)=(AB)/(PQ)`..............................(1)
Since, AX and PY are medians so they will divide their opposite sides.

or, `BX=(BC)/(2) and QY=(QR)/(2)`

Therefore, we have:

`(AB)/(PQ)= (BX)/(QY)`
`∠B = ∠Q`
So, we had observed that two respective sides are in same proportion in both triangles and also
angle included between them is respectively equal.
Hence, ∆ABX ~ ∆PQY (by SAS similarity rule)

`So,(AB)/(PQ)=(AX)/(PY)....................................(2)`
From (1) and (2),
`(AM)/(PN)=(AX)/(PY)`