Advertisements
Advertisements
Question
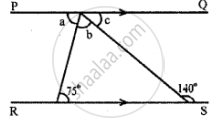
In the following figures, PQ is parallel to RS. Find the angles a, b and c:
Solution
In the given figure,
PQ || RS, ∠B = 75°, ∠ACS = 140°
AB is its transversal
∴ ∠PAB = ∠ABC
⇒ a = 75°
Again PQ || RS and AC is its transversal
∴ ∠QAC + ∠ACS = 180° (Co-interior angles)
⇒ c + 140° = 180°
⇒ c = 180° - 140° = 40°
But a + b + c = 180° (Straight line angles)
∴ 75° + b + 40° = 180°
⇒ b + 115° = 180°
⇒ b = 180° - 115° = 65°
Hence a = 75°, b = 65°, c = 40°
APPEARS IN
RELATED QUESTIONS
In the figure given below, find the measure of the angles denoted by x,y, z,p,q and r.

Construct the 90° angle, using ruler and a pair of compass only.
Construct the 120° angle, using ruler and a pair of compass only.
Construct the 135° angle, using ruler and a pair of compass only.
Draw a line segment AB = 7cm. Mark a point Pon AB such that AP=3 cm. Draw perpendicular on to AB at point P.
Draw a line segment AB = 6.5 cm. Locate a point P that is 5 cm from A and 4.6 cm from B. Through the point P, draw a perpendicular on to the line segment AB.
Draw a line segment OP = 8cm. Use set-square to construct ∠POQ = 90°; such that OQ = 6 cm. Join P and Q; then measure the length of PQ.
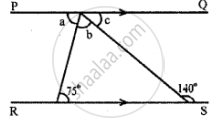
In the following figures, PQ is parallel to RS. Find the angles a, b and c:

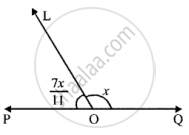
In the case given below, find the value of x so that POQ is straight line
Draw a line segment AB = 6 cm. Without using set squares, draw angle OAB = 60° and angle OBA = 90°. Measure angle AOB and write this measurement.
