Question

In a village, a well with 10 m inside diameter, is dug 14 m deep. Earth taken out of it is spread all around to a width 5 m to form an embankment. Find the height of the embankment. What value of the villagers is reflected here?

Answer 1

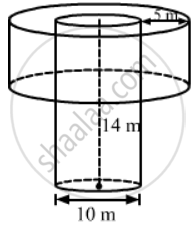
We have,

radius of well, `R = 10/2 = 5  "m"`

Depth of the well, H = 14 m and 

width of the embarkment = 5 m,

Also, the outer radius of the embankment, r = R + 5 + 5 + 5 = 10 m

And, the inner radius of the embarkment = R = 5 m

Let the height of the embarkment be h.

Now,

Volume of the embarkment = Volume of the earth taken out 

⇒ Volume of the embarkment = Volume of the well 

`rArr (pir^2 - pi "R"^2)"h" = pi"R"^2"H"`

`rArr pi (r^2 - "R"^2)"h" = pi"R"^2"H" `

`rArr (r^2 - "R"^2) "h" = "R"^2"H"`

`rArr (10^2 - 5^2)"h"= 5xx5xx14`

`rArr (100 - 25) "h" = 25 xx 14`

`rArr 75  "h" = 25xx14`

`rArr "h" = (25xx14)/75`

`therefore "h"= 14/3 "m"`

So, the height of the embankment is `14/3` m.

Value: We must lanour hard to make maximum use of the available resources.

Disclaimer: The answer provided in the textbook is incorrect. It has been corrected above.