Question

`phi` is the angle of the incline when a block of mass m just starts slipping down. The distance covered by the block if thrown up the incline with an initial speed u₀ is

Options

• `mu_0^2/(4g sin phi)`

• `(4mu_0^2)/(g sin phi)`

• `mu_0^2/((sin phi)/(4g))`

• `(4u_0^2 sin phi)/g`

Answer 1

`mu_0^2/(4g sin phi)`

Explanation:

Here `phi` is the angle of repose.

∴ `mu = tan phi`

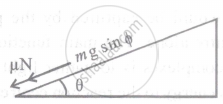
The retardation of the block

`a = F/m = (muN + mg sin phi)/m`

= `(tan phi xx mg cos phi + mg sin phi)/m`

= `2g sin phi`

 Let s is the distance travelled, then by third equation of motion

`v^2 = mu_0^2 = 2  a  s`

or 0 = `mu_0^2 - 2 xx 2g sin phi xx s` or s = `(mu_0^2)/(4g sin phi)`