Advertisements
Advertisements
Question
M and N are the mid-points of two equal chords AB and CD respectively of a circle with centre O. prove that:
(i) ∠BMN = ∠DNM.
(ii) ∠AMN = ∠CNM.
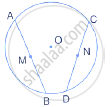
Sum
Solution
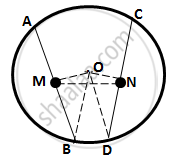
Drop OM⊥AB and ON⊥CD
∴ OM bisects AB and ON bisects CD
(Perpendicular drawn from the centre of a circle to a chord bisects it)
⇒ BM =`1/2` AB = `1/2` CD =DN .............(1)
Applying Pythagoras theorem,
OM2= OB2 -BM2
= OD2 - DN2 (by (1))
= ON2
∴ OM=ON
⇒ ∠OMN = ⇒ ∠ONM ……………(2)
(Angles opp to equal sides are equal)
(i) ∠OMB=∠OND (both 90°)
Subtracting (2) from above,
∠BMN= ∠DNM
(ii) ∠OMA = ONC (both 90°)
Adding (2) to above,
∠AMN = ∠CNM
shaalaa.com
Is there an error in this question or solution?
