Question

PA and PB are tangents from P to the circle with centre O. At point M, a tangent is drawn cutting PA at K and PB at N. Prove that KN = AK + BN.

Answer 1

We know that the tangents drawn from an external point to a circle are equal in length.

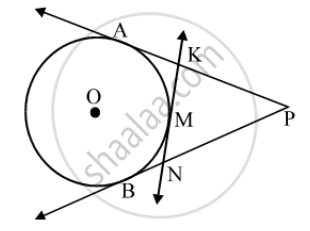
∴ PA = PB …. (i) [From P]

KA = KM …. (ii) [From K] and, NB = NM …. (iii) [From N]

Adding equations (ii) and (iii), we get

KA + NB = KM + NM

⇒ AK + BN = KM + MN ⇒ AK + BN = KN