Advertisements
Advertisements
Question
Show graphically that each one of the following systems of equations is inconsistent (i.e. has no solution) :
x − 2y = 6
3x − 6y = 0
Solution
The given equations are
x − 2y = 6 .....(i)
3x − 6y = 0 ....(ii)
Putting x = 0 in equation (i) we get
`=> 0 - 2y = 6`
`=> y = -3`
=> x = 0, y = -3
Putting y = 0 in equation (i) we get
`=> x - 2 xx 0 = 6`
=> x = 6
=> x = 6, y = 0
Use the following table to draw the graph.
| x | 0 | 6 |
| y | -3 | 0 |
The graph of (i) can be obtained by plotting the two points A(0, -3), B(6,0)
Graph of the equation….(ii)
3x - 6y = 0 ....(ii)
Putting x = 0 in equation (ii) we get
`=> 3 xx 0 - 6y = 0`
y = 0
x = 0, y = 0
Putting y = 1 in equation (ii) weget
`=> 3x - 6xx1 = 0`
=> x = 2
`=> x =2 , y = 1
Use the following table to draw the graph.
| x | 0 | 2 |
| y | 0 | 1 |
Draw the graph by plotting the two points C(0,0), D(2,1) from table
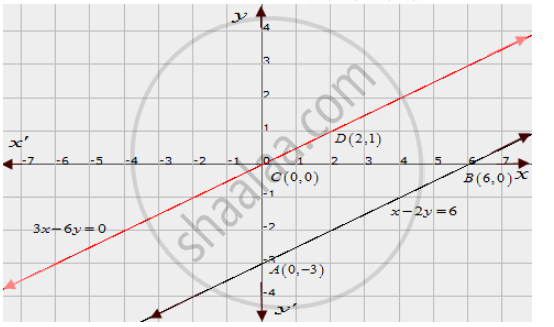
Here the two lines are parallel and so there is no point in common
Hence the given system of equations has no solution.
