Advertisements
Advertisements
Question
Solve the following inequalities and represent the solution on a number line:
2x + 3 < 5
Solution
We have, 2x + 3 < 5
⇒ 2x < 5 - 3
⇒ 2x < 2
⇒ x < 1
The graph of the solution set is {x < 1}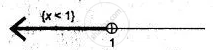
APPEARS IN
RELATED QUESTIONS
Solve the following inequation, write down the solution set and represent it on the real number line:
–2 + 10x ≤ 13x + 10 < 24 + 10x, x 𝜖 Z
Represent the solution of the following inequalities on the real number line:
4x – 1 > x + 11
Represent the solution of the following inequalities on the real number line:
x + 3 ≤ 2x + 9
Use the real number line to find the range of values of x for which:
–1 < x ≤ 6 and –2 ≤ x ≤ 3
Solve:
`(2x + 3)/3 >= (3x - 1)/4`, where x is a positive even integer
Solve the following linear in-equation and graph the solution set on a real number line :
`1/3 (2x - 1) < 1/4 (x + 5) < 1/6 (3x + 4)`, x ∈ R
Graph the solution set for each inequality:
x < 4
Graph the solution set for each inequality:
5 ≤ x < 10
If x ∈ W, find the solution set of `(3)/(5)x - (2x - 1)/(1) > 1` Also graph the solution set on the number line, if possible.
The solution set for the following number line is: