Advertisements
Advertisements
Question
Solve the following problem.
Derive the expression for power in terms of F, m, and t.
Solution
- A constant force F is applied to a body of mass (m) initially at rest (u = 0).
- We have, v = u + at
∴ v = 0 + at
∴ v = at ....(1) - Now, power is the rate of doing work,
∴ P = `"dW"/"dt"`
∴ P = `"F"*"ds"/"dt" ....[because "dW" = "F"*"ds"]` - But `"ds"/"dt" = "v"`, the instantaneous velocity of the particle.
∴ P = F·v ....(2) - According to Newton’s second law,
F = ma .....(3) - Substituting equations (1) and (3) in equation (2)
P = (ma)(at)
∴ P = ma2t
∴ P = `("m"^2"a"^2)/"m" xx "t"`
∴ P = `"F"^2/"m" "t"` - As F and m are constant, therefore, P ∝ t.
APPEARS IN
RELATED QUESTIONS
Answer the following question.
In the following table, every entry on the left column can match with any number of entries on the right side. Pick up all those and write respectively against A, B, C and D.
| Name of the force | Type of the force | ||
| A | Force due to tension in a string | P | EM force |
| B | Normal force | Q | Reaction force |
| C | Frictional force | R | Conservative force |
| D | Resistive force offered by air or water for objects moving through it. | S | Non-conservative force |
Answer the following question.
Distinguish between conservative and nonconservative forces.
Answer the following question.
State the formula for calculating work done by a force. Are there any conditions or limitations in using it directly? If so, state those clearly. Is there any mathematical way out for it? Explain.
Solve the following problem.
As I was standing on a weighing machine inside a lift it recorded 50 kg wt. Suddenly for a few seconds, it recorded 45 kg wt. What must have happened during that time? Explain with complete numerical analysis.
40000 litre of oil of density 0.9 g/cc is pumped from an oil tanker ship into a storage tank at 10 m higher level than the ship in half an hour. What should be the power of the pump?
Solve the following problem.
Power is the rate of doing work or the rate at which energy is supplied to the system. A constant force F is applied to a body of mass m. Power delivered by the force at time t from the start is proportional to ______.
Derive the expression for power in terms of F, m, and t.
Solve the following problem.
Two galaxies of masses 9 billion solar mass and 4 billion solar mass are 5 million light-years apart. If, the Sun has to cross the line joining them, without being attracted by either of them, through what point it should pass?
Solve the following problem.
While decreasing linearly from 5 N to 3 N, a force displaces an object from 3 m to 5 m. Calculate the work done by this force during this displacement.
Solve the following problem.
In the following table, every item on the left side can match with any number of items on the right-hand side. Select all those.
| Types of collision | Illustrations | ||
| a. | Elastic collision | i. | A ball hit by a bat. |
| b. | Inelastic collision | ii. | Molecular collisions responsible for pressure exerted by a gas. |
| c. | Perfectly inelastic collision | iii. | A stationary marble A is hit by marble B and the marble B comes to rest. |
| d. | Head-on collision | iv. | A blob of clay dropped on the ground sticks to the ground. |
| v. | Out of anger, giving a kick to a wall. | ||
| vi. | A striker hits the boundary of a carrom board in a direction perpendicular to the boundary and rebounds. | ||
Two spheres of masses m and M are situated in air and the gravitational force between them is F. The space around the masses is now filled with a liquid of specific gravity 3. The gravitational force will now be ______
Two bodies A and B of equal mass are suspended from two separate massles springs of force constant k1 and k2, respectively. The bodies oscillate vertically such that their maximum velocities are equal. The ratio of the amplitudes of body A to that of body B is ______.
In air, a charged soap bubble of radius 'R' breaks into 27 small soap bubbles of equal radius 'r '. Then the ratio of mechanical force acting per unit area of big soap bubble to that of a small soap bubble is ______.
Two particles of mass m1 and m2, approach each other due to their mutual gravitational attraction only. Then ______.
A body of mass 'm' begins to move under the action of time-dependent force `vec"F" = ("t"hat"i" + 2"t"^2hat"j")`N where `hat"i" and hat"j"` are unit vectors along x and y axis respectively. The power developed by the force in watt at time 't' is ______.
A force F = (10 + 0.5 x) N acts on a particle in the x-direction. The work done by the force in displacing the particle from x = 0 to x = 2 metre is ______.
Work done in sliding a 1 kg block up a rough inclined plane of height 5 m is 100 J. Work done against the friction is ______.
(g = 10 m/s2)
A Diwali cracker releases 25 gram gas per second, with a speed of 400 ms-1 after explosion. The force exerted by gas on the cracker is ______.
'n' number of balls each having mass 'm' and velocity 'u' hit a wall elastically and normally in 2 seconds. The force exerted by them on the wall is ______.
Out of the fundamental forces in nature, maximum and minimum range is respectively for ______.
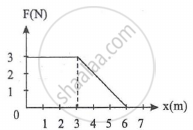
A force F acting on an object varies with distance x as shown here. The force is in N and x in m. The work done by the force in moving the object from x = 0 to x = 6 m is ______.
Two rods of same length and transfer a given amount of heat 12 second, when they are joined as shown in figure (i), But when they are joined as shown in figure (ii), then they will transfer same heat in same conditions in ______.

Which of the following statements is correct?
