Advertisements
Advertisements
Question
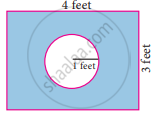
Some boys are playing a game, in which the stone thrown by them landing in a circular region is considered as win and landing other than the circular region is considered as a loss. What is the probability to win the game? (π = 3.14)
Solution
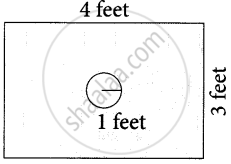
Area of a rectangle = l × b sq.feet
= 3 × 4 sq.feet
= 12 sq.feet
sample space (S) = 12
n(S) = 12
Let A be the event of getting the stone landing in a circular region
n(A) = Area of a circle
= πr2
= π × 1 × 1 ...(radius of a circle = 1 feet)
= π
P(A) = `("n"("A"))/("n"("S"))`
= `pi/12`
= `22/(7 xx 12)` or `3.14/12`
= `314/100`
= `157/600`
= `11/(7 xx 6)`
= `11/42`
Probability to win the game = `11/42` or `15/600`
APPEARS IN
RELATED QUESTIONS
If A is an event of a random experiment such that `"P"("A"):"P"(bar"A")` = 17 : 15 and n(S) = 640 then find `"P"(bar"A")`
A coin is tossed thrice. What is the probability of getting two consecutive tails?
Two unbiased dice are rolled once. Find the probability of getting a doublet (equal numbers on both dice)
A bag contains 5 red balls, 6 white balls, 7 green balls, 8 black balls. One ball is drawn at random from the bag. Find the probability that the ball drawn is not white
In a box there are 20 non-defective and some defective bulbs. If the probability that a bulb selected at random from the box found to be defective is `3/8` then, find the number of defective bulbs
Two customers Priya and Amuthan are visiting a particular shop in the same week (Monday to Saturday). Each is equally likely to visit the shop on any one day as on another day. What is the probability that both will visit the shop on different days?
Two customers Priya and Amuthan are visiting a particular shop in the same week (Monday to Saturday). Each is equally likely to visit the shop on any one day as on another day. What is the probability that both will visit the shop on consecutive days?
A page is selected at random from a book. The probability that the digit at units place of the page number chosen is less than 7 is
If a letter is chosen at random from the English alphabets {a, b, …, z}, then the probability that the letter chosen precedes x
A purse contains 10 notes of ₹ 2000, 15 notes of ₹ 500, and 25 notes of ₹ 200. One note is drawn at random. What is the probability that the note is either a ₹ 500 note or ₹ 200 note?
