Question

Read the following information carefully and answer the questions given below.

All the streets of a city are either perpendicular or parallel to one another. The streets are all straight. Streets N, O, P, Q and R are parallel to one another. Streets S, T, U, V, W, X and Y are horizontally parallel to one another,

(i) Street N is 1 km east of Street O.
(ii) Street O is 1/2 km west of Street P.
(iii) Street Q is 1 km west of Street R.
(iv) Street S is 1/2 km south of Street T.
(v) Street U is 1 km north of Street V.
(vi) Street W is 1/2 km north of Street X.
(vii) Street W is 1 km south of Street Y.

Street R is between O and P, then the distance between P and R is

Options

• `1/2` km

• 1 km

• 1.5 km

• 1.25 km

Answer 1

1.25 km

Explanation:

The vertical North-South streets are N, O, P, Q, R. From the basic information we have two relative positions are available - one between O, P and N and the other between Q and R.

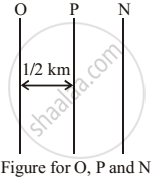
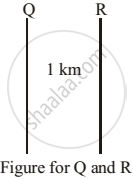
The horizontal east-west streets are S, T, U, V, W, X, Y. of these seven streets the relative positioning is given in 3 distinct part as shown here:

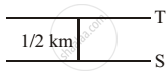
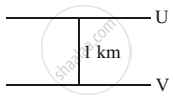
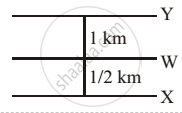
If R is placed between O and P. Q to N would be a minimum of 1.5 and a maximum of 2 kms. 
1.25 km is correct.