Question

The angle of elevation of the top of a tower from a point A due south of the tower is α and from B due east of the tower is β. If AB = d, show that the height of the tower is

`\frac{d}{\sqrt{\cot ^{2}\alpha +\cot^{2}\beta `

Answer 1

Let OP be the tower and let A and B be two points due south and east respectively of the tower such that ∠OAP = αand ∠OBP = β.

Let OP = h. In ∆OAP, we have

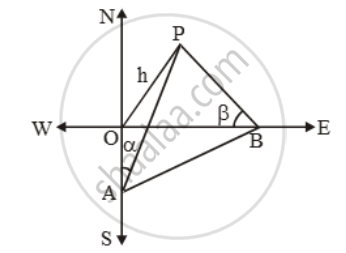
`\tan \alpha =\frac{h}{OA}`

⇒ OA = h cot α ….(i)

In ∆OBP, we have

`\tan \beta =\frac{h}{OB}`

⇒ OB = h cot β. ….(ii)

Since OAB is a right angled triangle. Therefore,

`AB^2 = OA^2 + OB^2`

`⇒ d^2 = h^2 cot^2 α + h^2 cot^2 β`

`\Rightarrow h=\frac{d}{\sqrt{\cot ^{2}\alpha +\cot ^{2}\beta )`

[Using (i) and (ii)]